Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

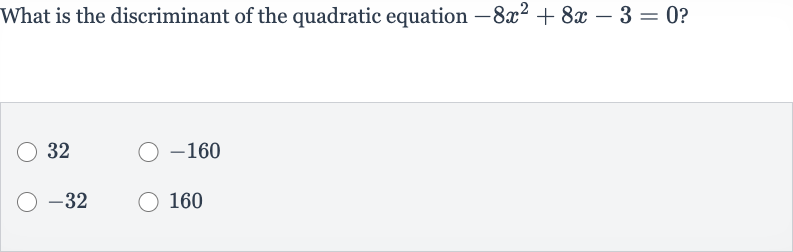
What is the discriminant of the quadratic equation
-8x^(2)+8x-3=0 ?
32

-160

-32
160

What is the discriminant of the quadratic equation $-8 x^{2}+8 x-3=0$ ? $\newline$ $32$ $\newline$ $-160$ $\newline$ $-32$ $\newline$ $160$

View step-by-step help

View step-by-step help

Division patterns with zeros

Full solution

Q. What is the discriminant of the quadratic equation

-8 x^{2}+8 x-3=0

?

\newline

32

\newline

-160

\newline

-32

\newline

160

Identify Coefficients: The discriminant of a quadratic equation in the form $ax^2 + bx + c = 0$ is given by the formula $D = b^2 - 4ac$ . We need to identify the coefficients $a$ , $b$ , and $c$ from the given equation.
Find Coefficients: In the equation $-8x^2 + 8x - 3 = 0$ , the coefficient $a$ is $-8$ , the coefficient $b$ is $8$ , and the coefficient $c$ is $-3$ .
Calculate Discriminant: Now we will calculate the discriminant using the formula $D = b^2 - 4ac$ . Substituting the values we get $D = (8)^2 - 4\cdot(-8)\cdot(-3)$ .
Substitute Values: Calculating the discriminant, we have $D = 64 - 4 \times 8 \times 3$ .
Simplify Calculation: Further simplifying, we get $D = 64 - 96$ .
Final Result: Finally, we find that $D = -32$ .

More problems from Division patterns with zeros

Question

\newline

90 \div 15 =

Posted 2 months ago

Question

A basketball game was scheduled to last

60

minutes, including the breaks between quarters. Several timeouts were used throughout the game, resulting in the game lasting a total of

72

minutes, including breaks. If each timeout was

90

seconds, how many total timeouts were used during the game?

Posted 4 months ago

Question

Floyd is an aspiring music artist. He has a record contract that pays him a base rate of

\$ 200

a month and an additional

\$ 12

for each album that he sells. Last month he earned a total of

\$ 644

. How many albums did Floyd sell last month?

Posted 1 month ago

Question

Of a squirrel's hidden nuts, for every

5

that get found, there are

3

that do not get found. A squirrel hid

40

nuts all together.

\newline

How many of the nuts do not get found?

\newline

Posted 1 month ago

Question

According to Greg, perfect cherry pies have a ratio of

240

3

\newline

How many cherries does Greg need to make

15

perfect cherry pies?

\newline

Posted 4 months ago

Question

A biologist puts a certain number of DNA strands into a machine, which uses a process called PCR cycling. Each PCR cycle, the machine creates a copy of every DNA strand. All of the DNA strands (the original DNA strands and their copies) remain together in the machine, ready for more PCR cycles. If a biologist puts

1

thousand DNA strands into the machine and runs it for

4

cycles, how many DNA strands will be in the machine at the end of the process, in thousands?

Posted 26 days ago

Question

Darius buys a bottle of a chemical solution that contains

70 \%

alcohol. The bottle contains

500

milliliters of solution. How many milliliters of alcohol are in the solution?

Posted 2 months ago

Question

Ana drinks horchata out of glasses that each hold

\frac{1}{8}

of a liter. She has

\frac{7}{10}

of a liter of horchata in her refrigerator.

\newline

How many glasses of horchata can she pour?

\newline

Posted 1 month ago

Question

6

loaves of elf bread on an

8

day hiking trip. He wants to know how many loaves of elf bread (b) he should pack for a

12

day hiking trip if he eats the same amount of bread each day.

\newline

How many loaves of elf bread should Sam pack for a

12

\newline

Posted 2 months ago

Question

Chef Rita is cooking for a Sunday brunch. She knows that

22

pancakes can feed

8

people. She is wondering how many people

(p)

she can feed with

55

pancakes. She assumes each person eats the same quantity of pancakes.

\newline

How many people can Rita feed with

55

\newline

Posted 3 months ago