AI tutor
Full solution
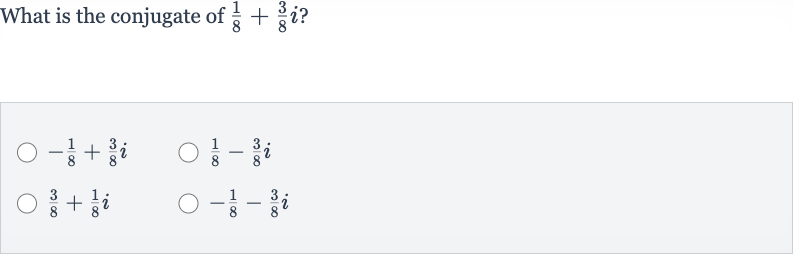
Q. What is the conjugate of ?
- Understand Complex Conjugate: Understand the concept of a complex conjugate. The conjugate of a complex number is , where and are real numbers.
- Identify Real and Imaginary Parts: Identify the real and imaginary parts of the given complex number.The given complex number is , where the real part is and the imaginary part is .
- Apply Conjugate Rule: Apply the conjugate rule to the given complex number.To find the conjugate, we change the sign of the imaginary part. Therefore, the conjugate of is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help