Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

What is the area of the region between the graphs of
f(x)=x^(2)+2x and
g(x)=2x+1 ?
Choose 1 answer:
(A)
(2)/(3)
(B) 2
(C)
(4)/(3)
(D)
(10)/(3)

What is the area of the region between the graphs of $f(x)=x^{2}+2 x$ and $g(x)=2 x+1$ ? $\newline$ Choose $1$ answer: $\newline$ (A) $\frac{2}{3}$ $\newline$ (B) $2$ $\newline$ (C) $\frac{4}{3}$ $\newline$ (D) $\frac{10}{3}$

View step-by-step help

View step-by-step help

Intermediate Value Theorem

Full solution

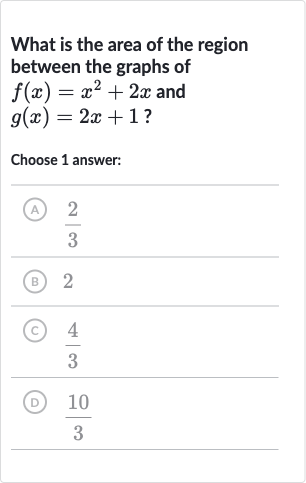
Q. What is the area of the region between the graphs of

f(x)=x^{2}+2 x

and

g(x)=2 x+1

?

\newline

Choose

1

answer:

\newline

(A)

\frac{2}{3}

\newline

(B)

2

\newline

(C)

\frac{4}{3}

\newline

(D)

\frac{10}{3}

Set Equations Equal: To find the area between the two curves, we first need to find the points of intersection by setting $f(x)$ equal to $g(x)$ .
Solve for x: Set $f(x) = g(x)$ : $x^2 + 2x = 2x + 1$ .
Calculate Limits of Integration: Subtract $2x$ from both sides to simplify the equation: $x^2 = 1$ .
Set up Integral: Take the square root of both sides to find the values of $x$ : $x = \pm 1$ .
Substitute Functions: The points of intersection are $x = -1$ and $x = 1$ . These will be the limits of integration to find the area between the curves.
Simplify Integrand: Set up the integral to calculate the area: $A = \int_{-1}^{1} (g(x) - f(x)) \, dx$ .
Integrate Function: Substitute the functions into the integral: $A = \int_{-1}^{1} ((2x + 1) - (x^2 + 2x)) \, dx$ .
Evaluate Upper Limit: Simplify the integrand: $A = \int_{-1}^{1} (1 - x^2) \, dx$ .
Evaluate Lower Limit: Integrate the function: $A = [x - (\frac{1}{3})x^3]$ from $-1$ to $1$ .
Find Total Area: Evaluate the integral at the upper limit: $A(1) = 1 - (\frac{1}{3})(1)^3 = 1 - \frac{1}{3} = \frac{2}{3}$ .
Final Answer: Evaluate the integral at the lower limit: $A(-1) = -1 - \frac{1}{3}(-1)^3 = -1 + \frac{1}{3} = -\frac{2}{3}$ .
Final Answer: Evaluate the integral at the lower limit: $A(-1) = -1 - (\frac{1}{3})(-1)^3 = -1 + \frac{1}{3} = -\frac{2}{3}$ .Find the difference between the two evaluations to get the total area: $A = A(1) - A(-1) = \frac{2}{3} - (-\frac{2}{3}) = \frac{2}{3} + \frac{2}{3} = \frac{4}{3}$ .
Final Answer: Evaluate the integral at the lower limit: $A(-1) = -1 - (1/3)(-1)^3 = -1 + 1/3 = -2/3$ .Find the difference between the two evaluations to get the total area: $A = A(1) - A(-1) = 2/3 - (-2/3) = 2/3 + 2/3 = 4/3$ .The area of the region between the graphs of $f(x)$ and $g(x)$ is $4/3$ , which corresponds to answer choice (C).

More problems from Intermediate Value Theorem

Question

f

be a continuous function on the closed interval

[1,5]

f(1)=1

f(5)=-3

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

f(c)=2

for at least one

c

-3

1

\newline

f(c)=-2

for at least one

c

-3

1

\newline

f(c)=2

for at least one

c

1

5

\newline

f(c)=-2

for at least one

c

1

5

Posted 3 months ago

Question

h

be a continuous function on the closed interval

[-3,4]

h(-3)=-1

h(4)=2

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

h(c)=1

for at least one

c

-3

4

\newline

h(c)=-2

for at least one

c

-3

4

\newline

h(c)=-2

for at least one

c

-1

2

\newline

h(c)=1

for at least one

c

-1

2

Posted 3 months ago

Question

g

be a continuous function on the closed interval

[-3,3]

g(-3)=0

g(3)=6

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

g(c)=-2

for at least one

c

-3

3

\newline

g(c)=-2

for at least one

c

0

6

\newline

g(c)=5

for at least one

c

-3

3

\newline

g(c)=5

for at least one

c

0

6

Posted 3 months ago

Question

g

be a continuous function on the closed interval

[-1,4]

g(-1)=-4

g(4)=1

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

g(c)=3

for at least one

c

-1

4

\newline

g(c)=-3

for at least one

c

-4

1

\newline

g(c)=3

for at least one

c

-4

1

\newline

g(c)=-3

for at least one

c

-1

4

Posted 3 months ago

Question

g

be a continuous function on the closed interval

[-1,3]

g(-1)=-2

g(3)=-5

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

g(c)=-3

for at least one

c

-5

-2

\newline

g(c)=0

for at least one

c

-5

-2

\newline

g(c)=0

for at least one

c

-1

3

\newline

g(c)=-3

for at least one

c

-1

3

Posted 3 months ago

Question

f

be a continuous function on the closed interval

[-2,1]

f(-2)=3

f(1)=6

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

f(c)=0

for at least one

c

-2

1

\newline

f(c)=0

for at least one

c

3

6

\newline

f(c)=4

for at least one

c

-2

1

\newline

f(c)=4

for at least one

c

3

6

Posted 3 months ago

Question

f

be a continuous function on the closed interval

[-5,0]

f(-5)=0

f(0)=5

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

f(c)=-2

for at least one

c

-5

0

\newline

f(c)=2

for at least one

c

0

5

\newline

f(c)=2

for at least one

c

-5

0

\newline

f(c)=-2

for at least one

c

0

5

Posted 3 months ago

Question

f

be a continuous function on the closed interval

[1,5]

f(1)=1

f(5)=-3

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

f(c)=2

for at least one

c

1

5

\newline

f(c)=-2

for at least one

c

-3

1

\newline

f(c)=-2

for at least one

c

1

5

\newline

f(c)=2

for at least one

c

-3

1

Posted 3 months ago

Question

h(x)=\frac{1}{x}

\newline

Can we use the intermediate value theorem to say the equation

h(x)=0.5

has a solution where

-1 \leq x \leq 1

\newline

1

\newline

(A) No, since the function is not continuous on that interval.

\newline

0

5

h(-1)

h(1)

\newline

(C) Yes, both conditions for using the intermediate value theorem have been met.

Posted 3 months ago

Question

h

be a continuous function on the closed interval

[0,4]

h(0)=2

h(4)=-2

\newline

Which of the following is guaranteed by the Intermediate Value Theorem?

\newline

1

\newline

h(c)=3

for at least one

c

0

4

\newline

h(c)=-1

for at least one

c

0

4

\newline

h(c)=-1

for at least one

c

-2

2

\newline

h(c)=3

for at least one

c

-2

2

Posted 3 months ago