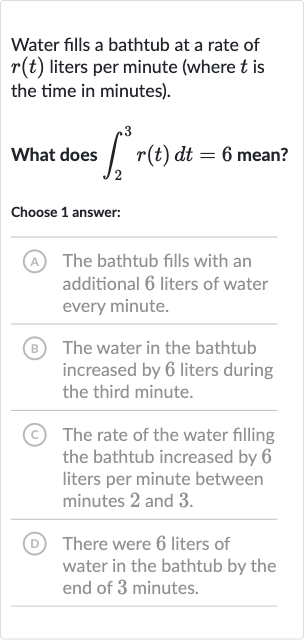
Water fills a bathtub at a rate of r(t) liters per minute (where t is the time in minutes). What does int_(2)^(3)r(t)dt=6 mean? Choose 1 answer: (A) The bathtub fills with an additional 6 liters of water every minute. (B) The water in the bathtub increased by 6 liters during the third minute. (C) The rate of the water filling the bathtub increased by 6 liters per minute between minutes 2 and 3 . (D) There were 6 liters of water in the bathtub by the end of 3 minutes.

Question

Water fills a bathtub at a rate of  r(t) liters per minute (where  t is the time in minutes). What does  int_(2)^(3)r(t)dt=6 mean? Choose 1 answer: (A) The bathtub fills with an additional 6 liters of water every minute. (B) The water in the bathtub increased by 6 liters during the third minute. (C) The rate of the water filling the bathtub increased by 6 liters per minute between minutes 2 and 3 . (D) There were 6 liters of water in the bathtub by the end of 3 minutes.

Bytelearn · Accepted Answer

Understand the integral: Understand the integral in the context of the problem. The integral of a rate function over a time interval gives the total amount of change over that interval. In this case, r(t) is the rate at which water fills the bathtub, and the integral from 2 to 3 of r(t) dt represents the total amount of water that has filled the bathtub from minute 2 to minute 3.Interpret the result: Interpret the result of the integral. Since the integral from 2 to 3 of r(t) dt equals 6, this means that from minute 2 to minute 3, the bathtub has been filled with 6 liters of water.Match interpretation to choices: Match the interpretation to the given choices. The correct interpretation is that the water in the bathtub increased by 6 liters during the time interval from minute 2 to minute 3. This matches choice (B) and not the other choices.

Full solution

More problems from Interpreting Linear Expressions

Related topics