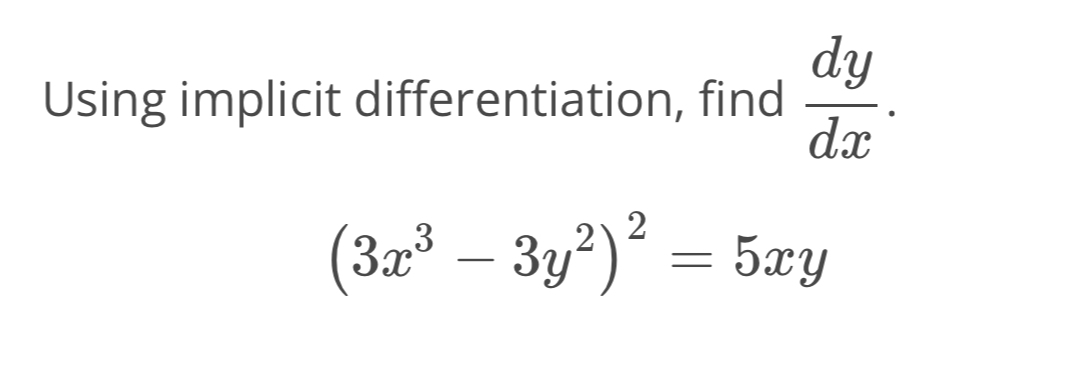Using implicit differentiation, find (dy)/(dx). (3x^(3)-3y^(2))^(2)=5xy

Question

Using implicit differentiation, find  (dy)/(dx).  (3x^(3)-3y^(2))^(2)=5xy

Bytelearn · Accepted Answer

Apply Chain Rule: First, we need to apply the chain rule to differentiate both sides of the equation with respect to x. The left side of the equation is a function raised to the second power, so we'll use the chain rule to differentiate it. The right side is a product of x and y, so we'll use the product rule to differentiate it.Differentiate Left Side: Differentiate the left side of the equation (3x^3 - 3y^2)^2 with respect to x. Using the chain rule, we get 2(3x^3 - 3y^2) * d/dx(3x^3 - 3y^2). Now we need to differentiate the inside function 3x^3 - 3y^2 with respect to x.Substitute Inside Function: Differentiate 3x^3 with respect to x to get 9x^2. Since y is a function of x, we need to use the chain rule to differentiate -3y^2 with respect to x, which gives us -6y * dy/dx. So the derivative of the inside function is 9x^2 - 6y * dy/dx.Differentiate Right Side: Now we substitute the derivative of the inside function back into the chain rule expression. We get 2(3x^3 - 3y^2) * (9x^2 - 6y * dy/dx).Set Equations Equal: Next, differentiate the right side of the equation 5xy with respect to x using the product rule. The derivative of 5xy with respect to x is 5y + 5x * dy/dx.Expand Left Side: Now we have the derivatives of both sides of the equation. Set them equal to each other to get 2(3x^3 - 3y^2) * (9x^2 - 6y * dy/dx) = 5y + 5x * dy/dx.Collect Terms: Expand the left side of the equation to simplify it. We get 2 * (27x^5 - 18x^2y * dy/dx - 27x^3y^2 + 18y^3 * dy/dx).Factor Out dy/dx: Distribute the 2 on the left side to get 54x^5 - 36x^2y * dy/dx - 54x^3y^2 + 36y^3 * dy/dx.Move Term: Now we need to collect all the terms with dy/dx on one side of the equation and the rest on the other side. We get -36x^2y * dy/dx + 36y^3 * dy/dx = 5y - 54x^5 + 54x^3y^2 - 5x * dy/dx.Solve for dy/dx: Factor out dy/dx on the left side of the equation to get dy/dx * (-36x^2y + 36y^3) = 5y - 54x^5 + 54x^3y^2 - 5x * dy/dx.Move the term -5x * dy/dx from the right side to the left side to get dy/dx * (-36x^2y + 36y^3 + 5x) = 5y - 54x^5 + 54x^3y^2.Now we can solve for dy/dx by dividing both sides of the equation by (-36x^2y + 36y^3 + 5x). We get dy/dx = (5y - 54x^5 + 54x^3y^2) / (-36x^2y + 36y^3 + 5x).

Using implicit differentiation, find $\frac{d y}{d x}$ . $\newline$ $\left(3 x^{3}-3 y^{2}\right)^{2}=5 x y$

Full solution

More problems from Simplify expressions using trigonometric identities

Related topics

Using implicit differentiation, find dydx \frac{d y}{d x} dxdy​.\newline(3x3−3y2)2=5xy \left(3 x^{3}-3 y^{2}\right)^{2}=5 x y (3x3−3y2)2=5xy

Using implicit differentiation, find $\frac{d y}{d x}$ . $\newline$ $\left(3 x^{3}-3 y^{2}\right)^{2}=5 x y$