Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The sum of two numbers is 17 . One number is 3 less than
(2)/(3) of the other number. What is the lesser number?
12
7
5
3
My Progress
Comerance

The sum of two numbers is $17$ . One number is $3$ less than $\frac{2}{3}$ of the other number. What is the lesser number? $\newline$ $12$ $\newline$ $7$ $\newline$ $5$ $\newline$ $3$ $\newline$

View step-by-step help

View step-by-step help

Round mixed numbers

Full solution

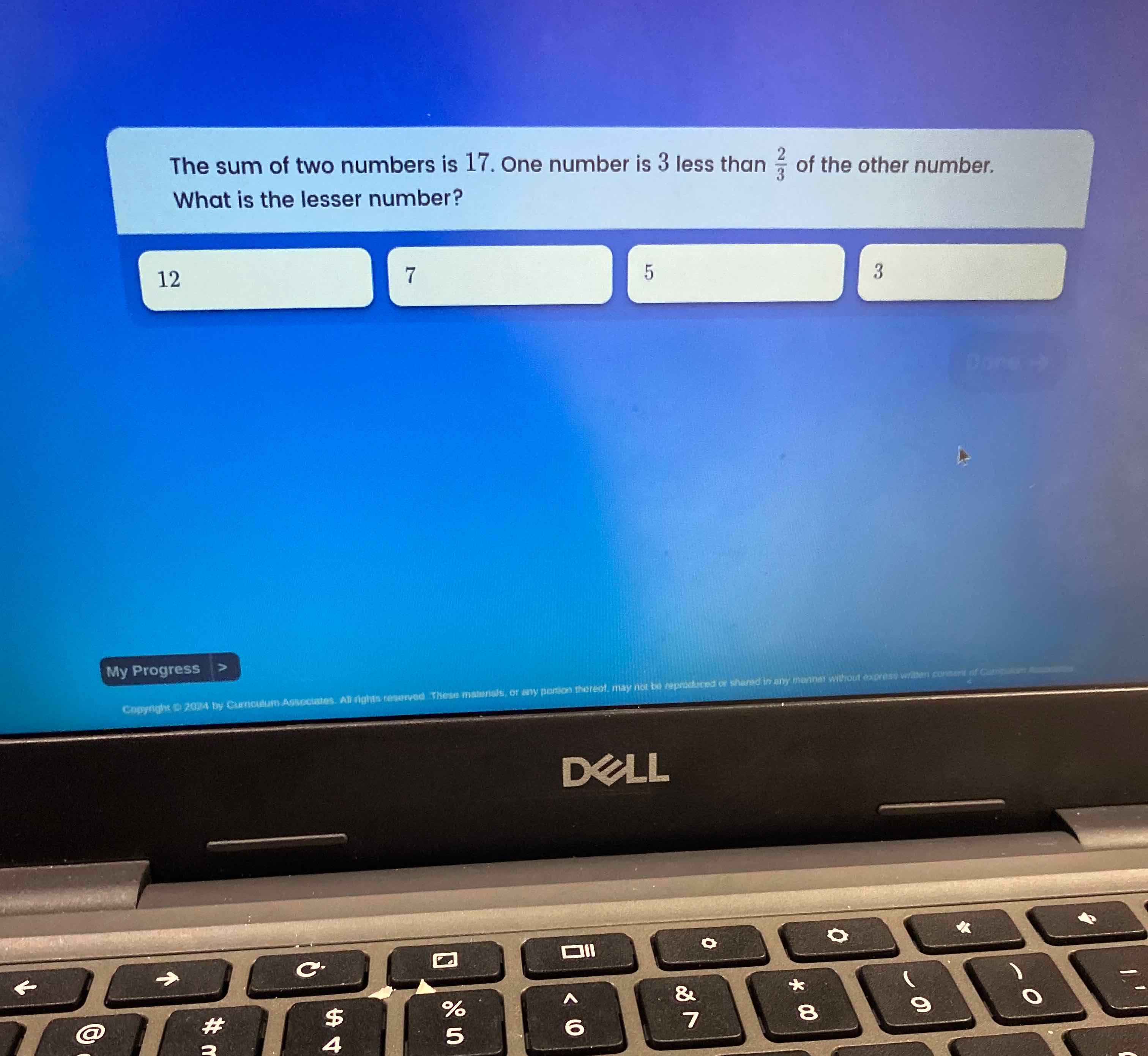
Q. The sum of two numbers is

17

. One number is

3

less than

\frac{2}{3}

of the other number. What is the lesser number?

\newline

12

\newline

7

\newline

5

\newline

3

\newline

Define Variables: Let $x$ be the larger number and $y$ be the smaller number. The sum of the two numbers is given as $17$ . So, we write the equation: $\newline$ $x + y = 17$
Express Relationship: It's also given that one number is $3$ less than two-thirds of the other number. We can express this relationship as: $y = \frac{2}{3}x - 3$
Substitute and Simplify: Substitute the expression for $y$ from the second equation into the first equation: $\newline$ $x + \frac{2}{3}x - 3 = 17$
Combine Like Terms: Combine like terms: $\frac{5}{3}x - 3 = 17$
Isolate $x$ : Solve for $x$ by first adding $3$ to both sides: $\newline$ $(\frac{5}{3})x = 20$
Find $y$ : Multiply both sides by $\frac{3}{5}$ to isolate $x$ :
$x = \left(\frac{3}{5}\right) \times 20$
$x = 12$
Final Solution: Substitute $x = 12$ back into the equation for $y$ : $y = \left(\frac{2}{3}\right)*12 - 3$ $y = 8 - 3$ $y = 5$

More problems from Round mixed numbers

Question

\frac{2}{6}

in lowest terms.

\newline

\underline{\hspace{1em}}

\underline{\hspace{1em}}

Posted 2 months ago

Question

Which fraction is greater?

\newline

\newline

\frac{8}{11}

\newline

\frac{7}{11}

Posted 2 months ago

Question

\frac{13}{5}

in mixed fraction.

Posted 2 months ago

Question

9

882

81

Posted 2 months ago

Question

Convert the following

\newline

\newline

165.078125_{(10)}

\newline

\newline

1101101101.10011_{(2)}

Posted 2 months ago

Question

\frac{9}{5}

into a mixed number.

Posted 2 months ago

Question

\frac{14}{5}

into a mixed number.

Posted 2 months ago

Question

\frac{24}{5}

into a mixed number.

Posted 2 months ago

Question

8 \frac{1}{6}

into an improper fraction.

\newline

Posted 2 months ago

Question

\frac{8}{5}

into a mixed number.

Posted 2 months ago