Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

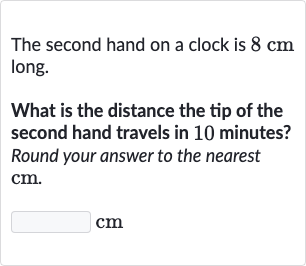
The second hand on a clock is 8cm long. What is the distance the tip of the second hand travels in 10 minutes? Round your answer to the nearest cm. cm

The second hand on a clock is 8 cm 8 \mathrm{~cm} long.\newlineWhat is the distance the tip of the second hand travels in 1010 minutes?\newlineRound your answer to the nearest cm \mathrm{cm} .\newline□\square cm \mathrm{cm}

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Geometryright chevron icon
Pythagorean theoremright chevron icon

Full solution

Q. The second hand on a clock is 8 cm 8 \mathrm{~cm} long.\newlineWhat is the distance the tip of the second hand travels in 1010 minutes?\newlineRound your answer to the nearest cm \mathrm{cm} .\newline□\square cm \mathrm{cm}
  1. Calculate Circumference Formula: The second hand makes a full circle every minute, so in 1010 minutes, it will make 1010 full circles.
  2. Calculate Radius Length: The circumference of a circle is given by the formula C=2⋅π⋅rC = 2 \cdot \pi \cdot r, where rr is the radius.
  3. Calculate Circumference: The length of the second hand is the radius, which is 88 cm. So, C=2×π×8C = 2 \times \pi \times 8.
  4. Multiply Circumference by Time: Calculate the circumference: C=2×π×8=16πC = 2 \times \pi \times 8 = 16\pi cm.
  5. Approximate Pi: Now, multiply the circumference by the number of circles made in 1010 minutes: 16π cm×10=160π cm16\pi \, \text{cm} \times 10 = 160\pi \, \text{cm}.
  6. Calculate Distance: Use the approximation π≈3.14\pi \approx 3.14 to find the distance: 160×3.14160 \times 3.14 cm.
  7. Round to Nearest cm: Calculate the distance: 160×3.14=502.4160 \times 3.14 = 502.4 cm.
  8. Round to Nearest cm: Calculate the distance: 160×3.14=502.4160 \times 3.14 = 502.4 cm.Round the answer to the nearest cm: 502.4502.4 cm ≈502\approx 502 cm.

More problems from Pythagorean theorem

Question
1919. Ms. Herbert has two rectangular bulletin boards in her classroom. Both bulletin boards have the same perimeter, but different areas. The first bulletin board is shown below. What could be the dimensions of the second bulletin board?\newlineC. \newline8ft×12ft8\text{ft}\times12\text{ft}\newlineD. \newline10ft×12ft10\text{ft}\times12\text{ft}
Get tutor helpright-arrow

Posted 2 months ago

Question
Roselyn is driving to visit her family, who live 150 kilometers150 \text{ kilometers} away. Her average speed is 60 kilometers per hour60 \text{ kilometers per hour}. The car's tank has 20 liters20 \text{ liters} of fuel at the beginning of the drive, and its fuel efficiency is 6 kilometers per liter6 \text{ kilometers per liter}. Fuel costs 0.60 dollars per liter0.60 \text{ dollars per liter}. \newlineHow long can Roselyn drive before she runs out of fuel?
Get tutor helpright-arrow

Posted 2 months ago

Question
A wet bicycle tire leaves a trace of water on the floor. The tire has a radius of 30 cm 30 \mathrm{~cm} , and the bicycle wheel makes 33 full rotations before stopping.\newlineHow long is the trace of water left on the floor?\newlineRound your answer to the nearest cm \mathrm{cm} .\newline□\square cm
Get tutor helpright-arrow

Posted 1 month ago

Question
Aliaa is skating down a ramp. The wheels on her skateboard have diameters of 60 mm 60 \mathrm{~mm} .\newlineWhat is the distance Aliaa travels when her skateboard wheels make 2020 revolutions?\newlineRound your answer to the nearest mm \mathrm{mm} .\newline□\square mm \mathrm{mm}
Get tutor helpright-arrow

Posted 1 month ago

Question
You are going on a field trip in a school bus. A special detector placed on one of the bus's tires counts the number of rotations completed by the tire during the journey. The tire's diameter is 11 meter, and it made 80008000 rotations during the trip.\newlineHow far did the bus travel?\newlineRound your answer to the nearest meter.\newline□\square meters
Get tutor helpright-arrow

Posted 1 month ago

Question
Bilal's fishing line was on a spool with a radius of 4 cm 4 \mathrm{~cm} . Suddenly, a fish pulled on the line, and the spool spun 1616 times before Bilal began to reel in the fish.\newlineWhat is the distance the fish pulled the fishing line?\newlineRound your answer to the nearest cm \mathrm{cm} .\newline□\square cm \mathrm{cm}
Get tutor helpright-arrow

Posted 1 month ago

Question
Isabella is playing with her yo-yo. The vertical distance Y Y (in cm \mathrm{cm} ) between the yo-yo and her hand t t seconds after she first spins it out is modeled by the following function. Here, t t is in radians.\newlineY(t)=40cos⁡(2π3t)−71 Y(t)=40 \cos \left(\frac{2 \pi}{3} t\right)-71 \newlineHow long does it take the yo-yo to fall all the way down from its peak, and then rise up to a vertical distance of −80 cm -80 \mathrm{~cm} ?\newlineRound your final answer to the nearest tenth of a second.\newline□\square seconds
Get tutor helpright-arrow

Posted 1 month ago

Question
A 3535 foot ladder is set against the side of a house so that it reaches up 2121 feet. If Elijah grabs the ladder at its base and pulls it 44 feet farther from the house, how far up the side of the house will the ladder reach now?
Get tutor helpright-arrow

Posted 8 days ago

Question
A boat leaves crab island searching for treasure and goes 1212 miles south, then turns and goes east for 1616 miles. How far away is the boat from where it started?
Get tutor helpright-arrow

Posted 8 days ago

Question
Determina el conjunto solución de:\newline1−2x−3x2<0 1-2 x-3 x^{2}<0
Get tutor helpright-arrow

Posted 22 hours ago

View all (10)