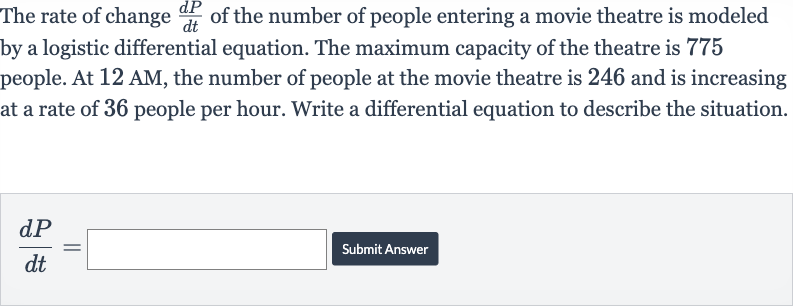
The rate of change (dP)/(dt) of the number of people entering a movie theatre is modeled by a logistic differential equation. The maximum capacity of the theatre is 775 people. At 12AM, the number of people at the movie theatre is 246 and is increasing at a rate of 36 people per hour. Write a differential equation to describe the situation. (dP)/(dt)=◻" Submit Answer "

Question

The rate of change  (dP)/(dt) of the number of people entering a movie theatre is modeled by a logistic differential equation. The maximum capacity of the theatre is 775 people. At  12AM, the number of people at the movie theatre is 246 and is increasing at a rate of 36 people per hour. Write a differential equation to describe the situation.  (dP)/(dt)=◻" Submit Answer "

Bytelearn · Accepted Answer

Logistic Differential Equation: The logistic differential equation is generally given by the formula: $$ \frac{dP}{dt} = rP\left(1 - \frac{P}{K}\right) $$ where $ P $ is the population at time $ t $, $ r $ is the intrinsic growth rate, and $ K $ is the carrying capacity, which in this case is the maximum capacity of the theatre.Maximum Capacity of Theatre: We are given that the maximum capacity $ K $ of the theatre is 775 people. This is the carrying capacity in our logistic model.Initial Condition: We are also given that at 12 AM, the number of people $ P $ is 246 and is increasing at a rate of 36 people per hour. This gives us the initial condition for $ P $ and the rate of change $ \frac{dP}{dt} $ at that specific time.Finding Intrinsic Growth Rate: To find the intrinsic growth rate $ r $, we use the given rate of change when $ P = 246 $. Plugging these values into the logistic equation, we get: $$ 36 = r \cdot 246 \left(1 - \frac{246}{775}\right) $$ Now we solve for $ r $.Calculating Fraction: First, calculate the fraction of the carrying capacity: $$ 1 - \frac{246}{775} = 1 - 0.3174 = 0.6826 $$Solving for r: Now, solve for $ r $: $$ 36 = r \cdot 246 \cdot 0.6826 $$ $$ r = \frac{36}{246 \cdot 0.6826} \approx \frac{36}{167.7996} \approx 0.2145 \text{ per hour} $$Complete Logistic Differential Equation: Now that we have the value for $ r $, we can write the complete logistic differential equation: $$ \frac{dP}{dt} = 0.2145P\left(1 - \frac{P}{775}\right) $$ This is the differential equation that describes the situation.

Full solution

More problems from Interpreting Linear Expressions

Related topics