Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The power generated by an electrical circuit (in watts) as a function of its current x (in amperes) is modeled by P(x)=-15 x(x-8) What current will produce the maximum power? ◻ amperes

The power generated by an electrical circuit (in watts) as a function of its current \newlinexx (in amperes) is modeled by\newlineP(x)=−15x(x−8)P(x)=-15 x(x-8)\newlineWhat current will produce the maximum power?\newline□\square amperes

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Ratio and Quadratic equationright chevron icon

Full solution

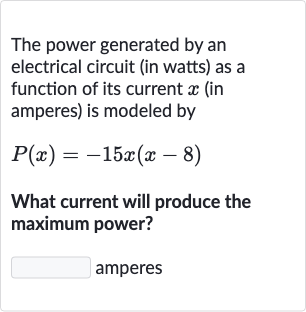
Q. The power generated by an electrical circuit (in watts) as a function of its current \newlinexx (in amperes) is modeled by\newlineP(x)=−15x(x−8)P(x)=-15 x(x-8)\newlineWhat current will produce the maximum power?\newline□\square amperes
  1. Analyze Quadratic Function: To find the current that will produce the maximum power, we need to analyze the quadratic function P(x)=−15x(x−8)P(x) = -15x(x - 8). This is a parabola that opens downwards because the coefficient of the x2x^2 term is negative (−15-15). The maximum power will be at the vertex of this parabola.
  2. Compare to General Form: The general form of a quadratic function is ax2+bx+cax^2 + bx + c. In our case, the function P(x)=−15x2+120xP(x) = -15x^2 + 120x can be compared to this form, where a=−15a = -15 and b=120b = 120. The xx-coordinate of the vertex of a parabola given by ax2+bx+cax^2 + bx + c is found using the formula −b/(2a)-b/(2a).
  3. Find x-coordinate of Vertex: We will apply the formula to find the x-coordinate of the vertex. For our function P(x)=−15x2+120xP(x) = -15x^2 + 120x, we have a=−15a = -15 and b=120b = 120. Plugging these values into the formula gives us x=−120(2⋅−15)x = \frac{-120}{(2 \cdot -15)}.
  4. Calculate x-coordinate: Calculating the x-coordinate of the vertex, we get x=−120(2⋅−15)=−120−30=4x = \frac{-120}{(2 \cdot -15)} = \frac{-120}{-30} = 4.
  5. Current for Maximum Power: The xx-coordinate of the vertex, which is 44 amperes, represents the current that will produce the maximum power in the electrical circuit.

More problems from Ratio and Quadratic equation

Question
Solve by completing the square.\newlinem2−10m−29=0m^2 - 10m - 29 = 0\newlineWrite your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.\newline`m` = ____ or `m` = _____
Get tutor helpright-arrow

Posted 1 month ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 5 5 units up of f(x)=x2 f(x)=x^2 .\newlineWrite your answer in the form a(x–h)2+k a(x–h)^2+k , where a a , h h , and k k are integers.\newlineg(x)= g(x)= ____
Get tutor helpright-arrow

Posted 1 month ago

Question
What is the range of this quadratic function?\newliney=x2−4x+4y = x^2 - 4x + 4\newlineChoices:\newline{y∣y≥2}\left\{y \mid y \geq 2\right\}\newline{y∣y≤0}\left\{y \mid y \leq 0\right\}\newline{y∣y≥0}\left\{y \mid y \geq 0\right\}\newlineall real numbers\text{all real numbers}
Get tutor helpright-arrow

Posted 1 month ago

Question
Write the equation of the parabola that passes through the points (1,0)(1,0), (2,0)(2,0), and (3,–16)(3,\text{–}16). Write your answer in the form y=a(x–p)(x–q)y = a(x – p)(x – q), where aa, pp, and qq are integers, decimals, or simplified fractions.\newline______
Get tutor helpright-arrow

Posted 1 month ago

Question
Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.\newlinef2+8f+_____f^2 + 8f + \_\_\_\_\_
Get tutor helpright-arrow

Posted 1 month ago

Question
Solve for h h .\newlineh2+39h=0 h^2 + 39h = 0 \newline\newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.\newlineh= h = ____\newline
Get tutor helpright-arrow

Posted 1 month ago

Question
Write a quadratic function with zeros −9-9 and −7-7.\newlineWrite your answer using the variable xx and in standard form with a leading coefficient of 11.\newlinef(x)=_____f(x) = \_\_\_\_\_
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the equation of the axis of symmetry for the parabola y=x2y = x^2. \newlineSimplify any numbers and write them as proper fractions, improper fractions, or integers.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 88 units up of f(x)=x2f(x) = x^2.\newlineWrite your answer in the form a(x–h)2+ka(x – h)^2 + k, where aa, hh, and kk are integers.\newlineg(x)=g(x) = ______\newline
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve for xx.\newlinex2=1x^2 = 1\newline\newlineWrite your answer in simplified, rationalized form.\newlinex=x = ______ or x=x = ______\newline
Get tutor helpright-arrow

Posted 2 months ago

View all (42)