Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

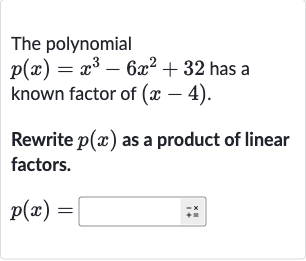
The polynomial p(x)=x^(3)-6x^(2)+32 has a known factor of (x-4).
Rewrite p(x) as a product of linear factors.
p(x) = ◻

The polynomial $p(x)=x^{3}-6 x^{2}+32$ has a known factor of $(x-4)$ . $\newline$ Rewrite $p(x)$ as a product of linear factors. $\newline$ $p(x) = \square$

View step-by-step help

View step-by-step help

Transformations of absolute value functions

Full solution

Q. The polynomial

p(x)=x^{3}-6 x^{2}+32

has a known factor of

(x-4)

.

\newline

Rewrite

p(x)

as a product of linear factors.

\newline

p(x) = \square

Factor Out Known Factor: Factor out the known factor from the polynomial. $\newline$ Since we know that $(x - 4)$ is a factor of $p(x)$ , we can perform polynomial division or use synthetic division to divide $p(x)$ by $(x - 4)$ to find the other factors.
Perform Synthetic Division: Perform the synthetic division using the known factor $(x - 4)$ . We set up the synthetic division with the root of the known factor, which is $4$ , and the coefficients of $p(x)$ , which are $1$ , $-6$ , and $32$ . $\newline$ $\newline$ $4$ | $1$ $-6$ $0$ $32$ $\newline$ | $4$ $4$ $2$ $4$ $3$ $\newline$ ----------------- $\newline$ $1$ $4$ $5$ $4$ $2$ $0$ $\newline$ The result of the synthetic division gives us the coefficients of the quotient polynomial: $4$ $8$ .
Factor Quotient Polynomial: Factor the quotient polynomial. $\newline$ The quotient polynomial is a quadratic, which we can factor further if possible. We look for two numbers that multiply to $-8$ and add up to $-2$ . These numbers are $-4$ and $2$ .
Write Factored Form: Write the factored form of the quotient polynomial. $\newline$ The factored form of the quadratic is $(x - 4)(x + 2)$ . Therefore, we can write $p(x)$ as the product of its factors: $\newline$ $p(x) = (x - 4)(x - 4)(x + 2)$ .
Simplify Repeated Factor: Simplify the repeated factor. $\newline$ Since $(x - 4)$ is a factor that appears twice, we can write it as a squared term: $\newline$ $p(x) = (x - 4)^2(x + 2)$ .

More problems from Transformations of absolute value functions

Question

What is the domain of this function?

\newline

(9,–2)(6,–10)(–3,9)(5,2)

\newline

\newline

(A)\{–3,5,6,9\}\newline(B)\{–10,–2,2,9\}\newline(C)\{2,5,6,9\}\newline(D)\{–6,–3,5,9\}

Posted 2 months ago

Question

Look at this set of ordered pairs:

\newline

(2, 14)

\newline

(10, 12)

\newline

(3, -19)

\newline

Is this relation a function?

\newline

\newline

\text{[[yes]}

\text{[no]]}

Posted 2 months ago

Question

Use the following function rule to find

f(11)

\newline

f(x) = -10 - 7x

\newline

f(11) =

Posted 2 months ago

Question

Find the slope of the line

y = -3x - \frac{2}{5}

\newline

Simplify your answer and write it as a proper fraction, improper fraction, or integer.

\newline

\_\_\_\_\_

Posted 2 months ago

Question

A line has a slope of

3

and passes through the point

(-2,-10)

. Write its equation in slope-intercept form.

\newline

Write your answer using integers, proper fractions, and improper fractions in simplest form.

Posted 2 months ago

Question

What is the range of this function?

\newline

\newline

\newline

\text{all real numbers}

\newline

\{y \mid y \geq 0\}

\newline

\{y \mid y \leq 0\}

\newline

\{y \mid y > 0\}

Posted 3 months ago

Question

g(x)

g(x)

is the translation

1

f(x) = |x|

\newline

Write your answer in the form

a|x - h| + k

a

h

k

\newline

g(x) =

\newline

Posted 3 months ago

Question

What is the domain of this function?

\newline

(8,–1)(7,–11)(–4,10)(4,3)

\newline

\newline

(A)\{–4,4,7,8\}\newline

(B)\{–11,–1,3,10\}\newline

(C)\{3,4,7,8\}\newline

(D)\{–7,–4,4,8\}

Posted 4 months ago

Question

What is the domain of this function?

\newline

(10,\,–3)(2,\,–9)(–5,\,8)(3,\,1)

\newline

\newline

[\{–5,2,3,10\}][\{–9,\,–3,1,8\}][\{1,2,3,10\}][\{–10,\,–5,2,3\}]

Posted 2 months ago

Question

What is the domain of this function?

\newline

(1,–4)(9,–12)(–6,11)(7,5)

\newline

\newline\\(A)\{–6,1,7,9\}\newline

(B)\{–12,–4,5,11\}\newline

(C\{5,1,7,9\}\newline

(D)\{–9,–6,1,7\}

Posted 2 months ago