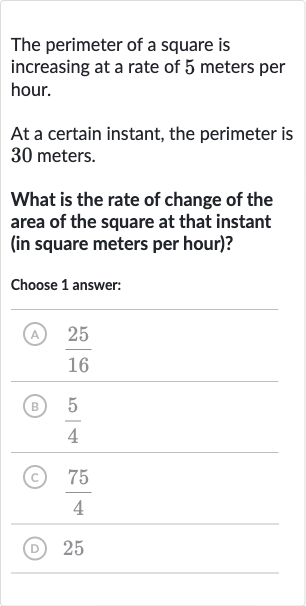
The perimeter of a square is increasing at a rate of $5$ meters per hour. $\newline$ At a certain instant, the perimeter is $30$ meters. $\newline$ What is the rate of change of the area of the square at that instant (in square meters per hour)? $\newline$ Choose $1$ answer: $\newline$ (A) $\frac{25}{16}$ $\newline$ (B) $\frac{5}{4}$ $\newline$ (C) $\frac{75}{4}$ $\newline$ (D) $25$

Full solution

Q. The perimeter of a square is increasing at a rate of

5

meters per hour.

\newline

At a certain instant, the perimeter is

30

meters.

\newline

What is the rate of change of the area of the square at that instant (in square meters per hour)?

\newline

Choose

1

answer:

\newline

(A)

\frac{25}{16}

\newline

(B)

\frac{5}{4}

\newline

(C)

\frac{75}{4}

\newline

(D)

25

Perimeter Calculation: The perimeter of a square is $4$ times the length of one side ( $s$ ), so if the perimeter ( $P$ ) is $30$ meters, then one side of the square is $\frac{30}{4}$ meters. $\newline$ $s = \frac{P}{4} = \frac{30}{4} = 7.5$ meters.
Area Differentiation: The area $A$ of a square is given by the formula $A = s^2$ . To find the rate of change of the area, we need to differentiate the area with respect to time $t$ . $\frac{dA}{dt} = 2s \cdot \frac{ds}{dt}$ .
Rate of Change Calculation: We know that the perimeter is increasing at a rate of $5$ meters per hour, so the rate of change of the side length $(\frac{ds}{dt})$ is $\frac{5}{4}$ meters per hour because the perimeter is $4$ times the side length. $\newline$ $\frac{ds}{dt} = \frac{dP}{dt} / 4 = \frac{5}{4}$ meters per hour.
Substitution of Values: Now we can substitute the values of $s$ and $\frac{ds}{dt}$ into the rate of change of the area formula. $\newline$ $\frac{dA}{dt} = 2 \times 7.5 \times \left(\frac{5}{4}\right) = 15 \times \left(\frac{5}{4}\right) = \frac{75}{4}$ square meters per hour.
Final Result: So, the rate of change of the area of the square at that instant is $\frac{75}{4}$ square meters per hour, which corresponds to answer choice (C).