Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

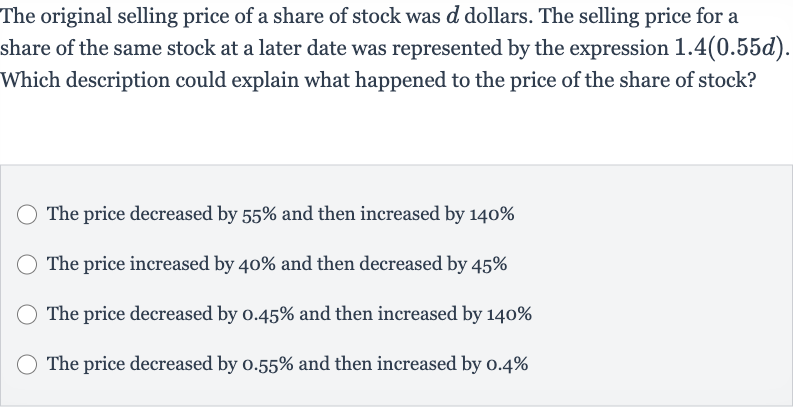
The original selling price of a share of stock was d dollars. The selling price for a share of the same stock at a later date was represented by the expression 1.4(0.55 d). Which description could explain what happened to the price of the share of stock? The price decreased by 55% and then increased by 140% The price increased by 40% and then decreased by 45% The price decreased by 0.45% and then increased by 140% The price decreased by 0.55% and then increased by 0.4%

The original selling price of a share of stock was d d dollars. The selling price for a share of the same stock at a later date was represented by the expression 1.4(0.55d) 1.4(0.55 d) . Which description could explain what happened to the price of the share of stock?\newlineThe price decreased by 55% 55 \% and then increased by 140% 140 \% \newlineThe price increased by 40% 40 \% and then decreased by 45% 45 \% \newlineThe price decreased by 0.45% 0.45 \% and then increased by 140% 140 \% \newlineThe price decreased by 0.55% 0.55 \% and then increased by 0.4% 0.4 \%

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Interpreting Linear Expressionsright chevron icon

Full solution

Q. The original selling price of a share of stock was d d dollars. The selling price for a share of the same stock at a later date was represented by the expression 1.4(0.55d) 1.4(0.55 d) . Which description could explain what happened to the price of the share of stock?\newlineThe price decreased by 55% 55 \% and then increased by 140% 140 \% \newlineThe price increased by 40% 40 \% and then decreased by 45% 45 \% \newlineThe price decreased by 0.45% 0.45 \% and then increased by 140% 140 \% \newlineThe price decreased by 0.55% 0.55 \% and then increased by 0.4% 0.4 \%
  1. Understand Expression: Understand the expression 1.4(0.55d)1.4(0.55d). The expression 1.4(0.55d)1.4(0.55d) can be broken down into two parts: 0.55d0.55d and 1.41.4. The 0.55d0.55d represents a 55%55\% decrease of the original price dd, because 0.550.55 is the same as 55100\frac{55}{100} or 55%55\%. The 1.41.4 represents a 1.4(0.55d)1.4(0.55d)11 increase of the price after the decrease, because 1.41.4 is the same as 1.4(0.55d)1.4(0.55d)33 or 1.4(0.55d)1.4(0.55d)44, which means the new price is 1.4(0.55d)1.4(0.55d)11 more than the price after the decrease.
  2. Calculate New Price: Calculate the new price after the initial decrease.\newlineTo find the new price after a 55%55\% decrease, we multiply the original price dd by 0.550.55. This gives us the price after the decrease.\newlineNew price after decrease = 0.55d0.55d
  3. Calculate Final Price: Calculate the final price after the subsequent increase.\newlineTo find the final price after a 40%40\% increase of the decreased price, we multiply the new price after decrease by 1.41.4.\newlineFinal price = 1.4×(0.55d)1.4 \times (0.55d)
  4. Interpret Price Change: Interpret the expression in terms of the price change.\newlineThe expression 1.4(0.55d)1.4(0.55d) represents a two-step price change. First, the price decreases by 55%55\%, and then the decreased price is increased by 40%40\%. This is the correct interpretation of the expression.

More problems from Interpreting Linear Expressions

Question
Simplify the expression:\newline7z2+3z27z^2 + 3z^2 \newline ______
Get tutor helpright-arrow

Posted 7 months ago

Question
Simplify the expression:\newline10f+8f−9f−6f10f + 8f - 9f - 6f\newline____
Get tutor helpright-arrow

Posted 7 months ago

Question
Subtract.\newline(3h+6)−(3h+2)(3h + 6) - (3h + 2)\newline____
Get tutor helpright-arrow

Posted 7 months ago

Question
Simplify the expression:\newline2p−p2p - p \newline______
Get tutor helpright-arrow

Posted 8 months ago

Question
A school charges $6\$6 per ticket to a dance. They spend $300\$300 on music and decorations. The expression 6n−3006n-300 describes the amount of profit the school makes from the dance if nn students buy tickets. What does 6n6n represent in this context?\newlineChoices:\newline(A)The amount of money the school charges for all `\n` tickets\newline(B)The amount of money the school spends on the dance\newline(C)The number of students who buy tickets\newline(D)The profit the school earns per ticket
Get tutor helpright-arrow

Posted 8 months ago

Question
Which is equal to cos⁡60∘ \cos 60^\circ ? \newlineChoices:\newline(A) sin⁡30∘ \sin 30^\circ \newline(B) sin⁡60∘ \sin 60^\circ \newline(C) cos⁡30∘ \cos 30^\circ \newline(D) cos⁡60∘ \cos 60^\circ
Get tutor helpright-arrow

Posted 9 months ago

Question
Which is equal to sin⁡45∘\sin 45^\circ? \newlineChoices:\newline(A) cos⁡45∘\cos 45^\circ\newline(B) cos⁡55∘\cos 55^\circ\newline(C) sin⁡55∘\sin 55^\circ\newline(D) sin⁡35∘\sin 35^\circ
Get tutor helpright-arrow

Posted 7 months ago

Question
Which is equal to cos⁡75∘\cos 75^\circ? \newlineChoices:\newline(A) sin⁡15∘\sin 15^\circ\newline(B) sin⁡75∘\sin 75^\circ\newline(C) cos⁡15∘\cos 15^\circ\newline(D) cos⁡25∘\cos 25^\circ
Get tutor helpright-arrow

Posted 7 months ago

Question
Which is equal to sin⁡30∘ \sin 30^\circ ? \newlineChoices:\newline(A) cos⁡60∘ \cos 60^\circ \newline(B) cos⁡30∘ \cos 30^\circ \newline(C) sin⁡60∘ \sin 60^\circ \newline(D) sin⁡30∘ \sin 30^\circ
Get tutor helpright-arrow

Posted 7 months ago

Question
Which is equal to cos⁡45∘ \cos 45^\circ ? \newlineChoices:\newline(A) sin⁡45∘ \sin 45^\circ \newline(B) sin⁡55∘ \sin 55^\circ \newline(C) cos⁡55∘ \cos 55^\circ \newline(D) cos⁡35∘ \cos 35^\circ
Get tutor helpright-arrow

Posted 7 months ago

View all (79)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant