Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

The graph of function
h is shown below. Let
g(x)=int_(1)^(x)h(t)dt.
Evaluate
g(4).

g(4)=

The graph of function $h$ is shown below. Let $g(x)=\int_{1}^{x} h(t) d t$ . $\newline$ Evaluate $g(4)$ . $\newline$ $g(4)=$

View step-by-step help

View step-by-step help

Evaluate rational expressions II

Full solution

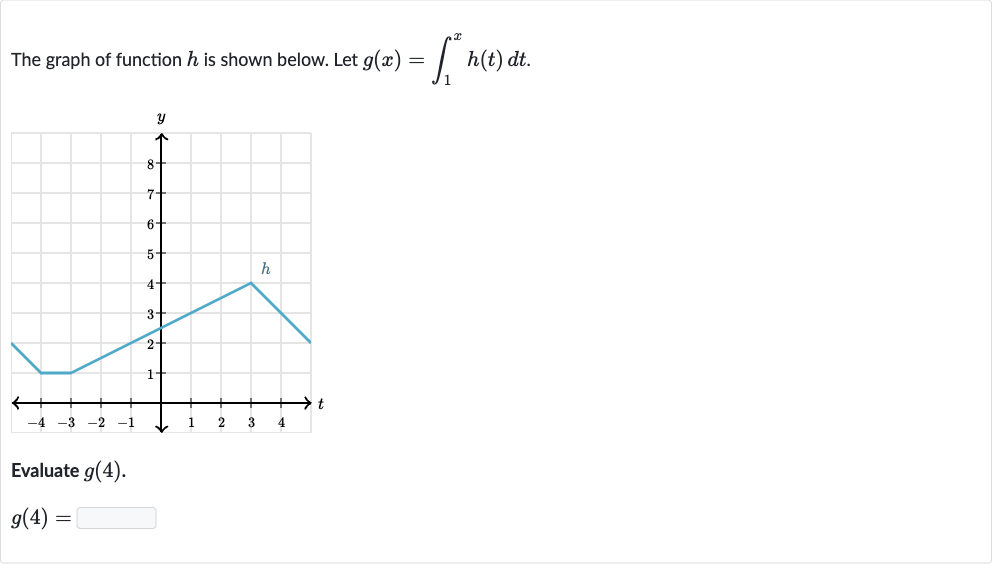
Q. The graph of function

h

is shown below. Let

g(x)=\int_{1}^{x} h(t) d t

.

\newline

Evaluate

g(4)

.

\newline

g(4)=

Substitute $x$ with $4$ : Substitute $x$ with $4$ in $g(x)$ to get $g(4)$ . $\newline$ $g(4) = \int_{1}^{4}h(t)\,dt$
Determine area under curve: Look at the graph of $h(t)$ to determine the area under the curve from $1$ to $4$ . Assuming the graph shows a rectangle from $1$ to $3$ with a height of $3$ , and a triangle from $3$ to $4$ with a base of $1$ and a height of $3$ .
Calculate rectangle area: Calculate the area of the rectangle. $\newline$ Area of rectangle = $\text{base} \times \text{height} = (3 - 1) \times 3 = 2 \times 3 = 6$
Calculate triangle area: Calculate the area of the triangle. $\newline$ Area of triangle = $(\text{base} \times \text{height}) / 2 = (1 \times 3) / 2 = 3 / 2 = 1.5$
Find $g(4)$ : Add the areas of the rectangle and triangle to find $g(4)$ . $\newline$ $g(4) = \text{Area of rectangle} + \text{Area of triangle} = 6 + 1.5 = 7.5$

More problems from Evaluate rational expressions II

Question

Divide. Write the quotient in lowest terms.

\newline

1 \frac{7}{9} \div \frac{4}{5}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

\frac{1}{4} \div 1 \frac{1}{8}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

1 \frac{1}{4} \div 1 \frac{2}{5}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

\frac{5}{8} \div 1 \frac{1}{3}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

4 \frac{4}{5} \div 1 \frac{1}{2}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

2 \frac{1}{2} \div 2 \frac{2}{3}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

3 \frac{3}{8} \div 9=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

3 \frac{3}{10} \div 1 \frac{4}{7}=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

4 \frac{2}{3} \div 7=

Posted 3 months ago

Question

Divide. Write the quotient in lowest terms.

\newline

3 \frac{1}{8} \div 1 \frac{2}{3}=

Posted 3 months ago