Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

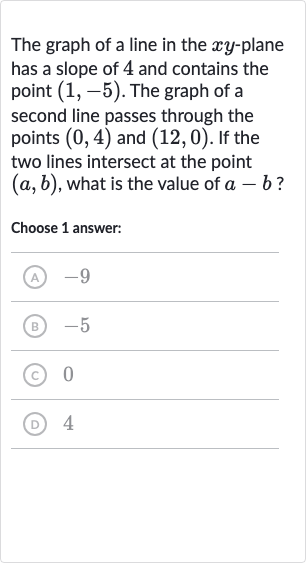
The graph of a line in the xy-plane has a slope of 4 and contains the point (1,-5). The graph of a second line passes through the points (0,4) and (12,0). If the two lines intersect at the point (a,b), what is the value of a-b ? Choose 1 answer: (A) -9 (B) -5 (C) 0 (D) 4

The graph of a line in the xyxy-plane has a slope of 44 and contains the point (1,−5)(1,-5). The graph of a second line passes through the points (0,4)(0,4) and (12,0)(12,0). If the two lines intersect at the point (a,b)(a,b), what is the value of a−ba-b?\newlineChoose 11 answer:\newline(A) −9-9\newline(B) −5-5\newline(C) 00\newline(D) 44

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Find a missing coordinate using sloperight chevron icon

Full solution

Q. The graph of a line in the xyxy-plane has a slope of 44 and contains the point (1,−5)(1,-5). The graph of a second line passes through the points (0,4)(0,4) and (12,0)(12,0). If the two lines intersect at the point (a,b)(a,b), what is the value of a−ba-b?\newlineChoose 11 answer:\newline(A) −9-9\newline(B) −5-5\newline(C) 00\newline(D) 44
  1. First Line Equation: The first line has a slope of 44 and passes through the point (1,−5)(1, -5). We can use the point-slope form to write the equation of the first line.\newliney−y1=m(x−x1)y - y_1 = m(x - x_1)\newliney−(−5)=4(x−1)y - (-5) = 4(x - 1)
  2. Second Line Equation: Simplify the equation of the first line.\newliney+5=4x−4y + 5 = 4x - 4\newliney=4x−4−5y = 4x - 4 - 5\newliney=4x−9y = 4x - 9\newlineThis is the equation of the first line.
  3. Calculate Second Line Slope: The second line passes through the points (0,4)(0, 4) and (12,0)(12, 0). We can find the slope of the second line using these points.\newlineSlope = (y2−y1)/(x2−x1)(y_2 - y_1) / (x_2 - x_1)\newlineSlope = (0−4)/(12−0)(0 - 4) / (12 - 0)
  4. Write Second Line Equation: Calculate the slope of the second line.\newlineSlope = −412-\frac{4}{12}\newlineSlope = −13-\frac{1}{3}\newlineThis is the slope of the second line.
  5. Find Intersection Point: Now we can write the equation of the second line using the point-slope form and one of the points, for example, (0,4)(0, 4).\newliney−y1=m(x−x1)y - y_1 = m(x - x_1)\newliney−4=(−13)(x−0)y - 4 = \left(-\frac{1}{3}\right)(x - 0)
  6. Solve for xx: Simplify the equation of the second line.y−4=(−13)xy - 4 = \left(-\frac{1}{3}\right)xy=(−13)x+4y = \left(-\frac{1}{3}\right)x + 4This is the equation of the second line.
  7. Calculate x-coordinate: To find the intersection point (a,b)(a, b), we set the equations of the two lines equal to each other.\newline4x−9=(−13)x+44x - 9 = (-\frac{1}{3})x + 4
  8. Calculate y-coordinate: Solve for xx by combining like terms.4x+(13)x=4+94x + (\frac{1}{3})x = 4 + 9(133)x=13(\frac{13}{3})x = 13
  9. Intersection Point Coordinates: Divide both sides by (13/3)(13/3) to solve for xx.x=13(13/3)x = \frac{13}{(13/3)}x=13×(313)x = 13 \times \left(\frac{3}{13}\right)x=3x = 3This is the xx-coordinate of the intersection point.
  10. Calculate a−ba - b: Now we substitute x=3x = 3 into one of the line equations to find the y-coordinate of the intersection point. We can use the first line's equation.\newliney=4x−9y = 4x - 9\newliney=4(3)−9y = 4(3) - 9
  11. Calculate a−ba - b: Now we substitute x=3x = 3 into one of the line equations to find the y-coordinate of the intersection point. We can use the first line's equation.\newliney=4x−9y = 4x - 9\newliney=4(3)−9y = 4(3) - 9Calculate the y-coordinate.\newliney=12−9y = 12 - 9\newliney=3y = 3\newlineThis is the y-coordinate of the intersection point.
  12. Calculate a−ba - b: Now we substitute x=3x = 3 into one of the line equations to find the y-coordinate of the intersection point. We can use the first line's equation.y=4x−9y = 4x - 9y=4(3)−9y = 4(3) - 9Calculate the y-coordinate.y=12−9y = 12 - 9y=3y = 3This is the y-coordinate of the intersection point.Now we have the intersection point (a,b)(a, b) which is (3,3)(3, 3). To find a−ba - b, we subtract the y-coordinate from the x-coordinate.a−b=3−3a - b = 3 - 3
  13. Calculate a−ba - b: Now we substitute x=3x = 3 into one of the line equations to find the y-coordinate of the intersection point. We can use the first line's equation.\newliney=4x−9y = 4x - 9\newliney=4(3)−9y = 4(3) - 9Calculate the y-coordinate.\newliney=12−9y = 12 - 9\newliney=3y = 3\newlineThis is the y-coordinate of the intersection point.Now we have the intersection point (a,b)(a, b) which is (3,3)(3, 3). To find a−ba - b, we subtract the y-coordinate from the x-coordinate.\newlinea−b=3−3a - b = 3 - 3Calculate a−ba - b.\newlinea−b=0a - b = 0\newlineThis is the value of a−ba - b.

More problems from Find a missing coordinate using slope

Question
What is the slope of the line through (−2,−6)(-2,-6) and (2,2)(2,2)?\newlineChoose 11 answer:\newline(A) 12\frac{1}{2}\newline(B) −2-2\newline(C) 22\newline(D) −12-\frac{1}{2}
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (−10,1)(-10,1) and (0,−4)(0,-4)? Choose 11 answer: \newline(A) 22 \newline(B) 12\frac{1}{2} \newline(C) −12-\frac{1}{2} \newline(D) −2-2
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (−9,−6)(-9,-6) and (3,−9)(3,-9)? Choose 11 answer: \newline(A) −4-4 \newline(B) 14\frac{1}{4} \newline(C) 44 \newline(D) −14-\frac{1}{4}
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through \newline(−5,−10)(-5,-10) and \newline(−1,5)(-1,5)?\newlineChoose 11 answer:\newline(A) \newline154\frac{15}{4}\newline(B) \newline415\frac{4}{15}\newline(C) \newline−154-\frac{15}{4}\newline(D) \newline−415-\frac{4}{15}
Get tutor helpright-arrow

Posted 8 months ago

Question
What is the slope of the line through (−1,8)(-1,8) and (3,−4)(3,-4)? Choose 11 answer: \newline(A) −13-\frac{1}{3} \newline(B) 13\frac{1}{3} \newline(C) 33 \newline(D) −3-3
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (−4,2)(-4,2) and (3,−3)(3,-3)? Choose 11 answer: (A) 75\frac{7}{5} (B) −75-\frac{7}{5} (C) −57-\frac{5}{7} (D) 57\frac{5}{7}
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (2,−2)(2,-2) and (9,3)(9,3)? \newlineChoose 11 answer:\newline(A) 57\frac{5}{7}\newline(B) −57-\frac{5}{7}\newline(C) 75\frac{7}{5}\newline(D) −75-\frac{7}{5}
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (1,−1)(1,-1) and (5,−7)(5,-7)? Choose 11 answer: (A) −32-\frac{3}{2} (B) 32\frac{3}{2} (C) −23-\frac{2}{3} (D) 23\frac{2}{3}
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (1,0)(1,0) and (3,8)(3,8)? Choose 11 answer: (A) −14-\frac{1}{4} (B) 44 (C) −4-4 (D) 14\frac{1}{4}
Get tutor helpright-arrow

Posted 10 months ago

Question
What is the slope of the line through (−1,−7)(-1,-7) and (3,9)(3,9)?\newlineChoose 11 answer:\newline(A) 14\frac{1}{4}\newline(B) 44\newline(C) −14-\frac{1}{4}\newline(D) −4-4
Get tutor helpright-arrow

Posted 10 months ago

View all (92)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant