Full solution
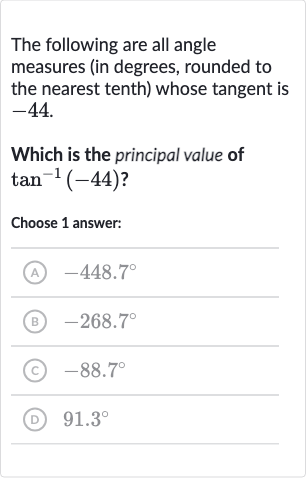
Q. The following are all angle measures (in degrees, rounded to the nearest tenth) whose tangent is .Which is the principal value of ?Choose answer:(A) (B) (C) (D)
- Finding the Inverse Tangent Function: The principal value of an inverse tangent function, , is the value of in the range -90° < \theta < 90° that satisfies . Since we are looking for , we need to find the angle whose tangent is and lies within the specified range.
- Using a Calculator or Computer: To find the principal value of , we can use a calculator or a computer to compute the inverse tangent of . Remember that the calculator should be set to degree mode since the answers are given in degrees.
- Calculating the Principal Value: Upon calculation, gives an angle of approximately degrees. This falls within the principal range of -90° < \theta < 90°.
- Determining the Final Answer: Therefore, the principal value of is degrees, which corresponds to option .
More problems from Solve trigonometric equations
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help