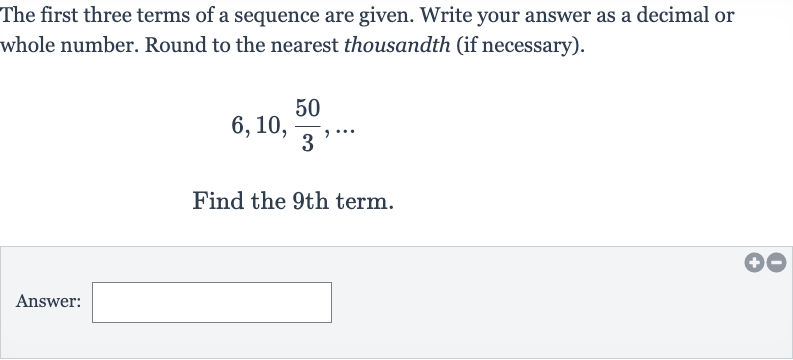
The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary). 6,10,(50)/(3),dots Find the 9th term. Answer:

Question

The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary).  6,10,(50)/(3),dots Find the 9th term. Answer:

Bytelearn · Accepted Answer

Identify Pattern: Identify the pattern in the sequence. The given sequence is 6, 10, 50/3, ... To find the pattern, we need to look at the differences or ratios between the terms. Let's check the differences first. Difference between second and first term: 10 - 6 = 4 Difference between third and second term: (50/3) - 10 = (50 - 30)/3 = 20/3 The differences are not constant, so this is not an arithmetic sequence. Let's check if it's a geometric sequence by finding the ratios. Ratio of second to first term: 10 / 6 = 5/3 Ratio of third to second term: (50/3) / 10 = (50/3) * (1/10) = 5/3 The ratios are constant, so this is a geometric sequence with a common ratio of 5/3.Use Formula for 9th Term: Use the formula for the nth term of a geometric sequence to find the 9th term. The formula for the nth term of a geometric sequence is a_n = a_1 * r^(n-1), where a_1 is the first term, r is the common ratio, and n is the term number. Here, a_1 = 6 (the first term), r = 5/3 (the common ratio), and n = 9 (since we're looking for the 9th term). Let's plug these values into the formula: a_9 = 6 * (5/3)^(9-1) a_9 = 6 * (5/3)^8Calculate 9th Term: Calculate the 9th term. a_9 = 6 * (5/3)^8 To simplify this, we can calculate (5/3)^8 first and then multiply by 6. (5/3)^8 = 5^8 / 3^8 = 390625 / 6561 Now, multiply this by 6: a_9 = 6 * (390625 / 6561) a_9 = 2343750 / 6561Simplify Fraction: Simplify the fraction to get the decimal value. a_9 = 2343750 / 6561 To get the decimal value, we divide 2343750 by 6561. a_9 ≈ 357.210 Since we need to round to the nearest thousandth, the 9th term is approximately 357.210.

The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary). $\newline$ $6,10, \frac{50}{3}, \ldots$ $\newline$ Find the $9$ th term. $\newline$ Answer:

Full solution

More problems from Find trigonometric functions using a calculator

Related topics

The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary).\newline6,10,503,… 6,10, \frac{50}{3}, \ldots 6,10,350​,…\newlineFind the 999th term.\newlineAnswer:

The first three terms of a sequence are given. Write your answer as a decimal or whole number. Round to the nearest thousandth (if necessary). $\newline$ $6,10, \frac{50}{3}, \ldots$ $\newline$ Find the $9$ th term. $\newline$ Answer: