Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

T=277+4s The temperature in kelvin, T, of a resistor in a circuit s seconds after connecting a battery is given by the equation. For how many seconds does the battery need to be connected for the temperature to increase by 1 kelvin? Choose 1 answer: (A) 0.25 (B) 4 (C) 69 (D) 277

T=277+4sT=277+4s\newlineThe temperature in kelvin, TT, of a resistor in a circuit ss seconds after connecting a battery is given by the equation. For how many seconds does the battery need to be connected for the temperature to increase by 11 kelvin?\newlineChoose 11 answer:\newline(A) 0.250.25\newline(B) 44\newline(C) 6969\newline(D) 277277

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Ratio and Quadratic equationright chevron icon

Full solution

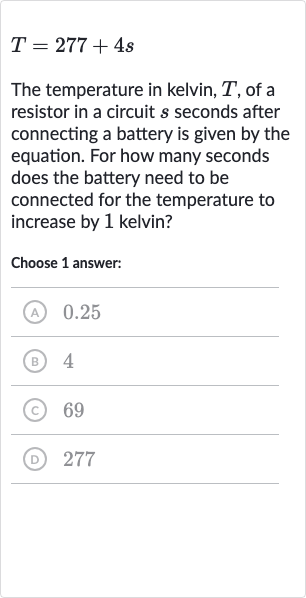
Q. T=277+4sT=277+4s\newlineThe temperature in kelvin, TT, of a resistor in a circuit ss seconds after connecting a battery is given by the equation. For how many seconds does the battery need to be connected for the temperature to increase by 11 kelvin?\newlineChoose 11 answer:\newline(A) 0.250.25\newline(B) 44\newline(C) 6969\newline(D) 277277
  1. Given Equation: We are given the equation T=277+4sT = 277 + 4s, where TT is the temperature in kelvin and ss is the time in seconds after connecting a battery. We want to find the value of ss that corresponds to an increase of 11 kelvin in temperature.
  2. Denote Initial Temperature: Let's denote the initial temperature as TinitialT_{\text{initial}}. After an increase of 11 kelvin, the new temperature will be Tinitial+1T_{\text{initial}} + 1. We can set up the equation Tinitial+1=277+4sT_{\text{initial}} + 1 = 277 + 4s to find the value of ss that corresponds to this increase.
  3. Set Up Equation: Since we are looking for the increase in temperature, we don't actually need to know the initial temperature. We can simply use the fact that the increase is 11 kelvin. So, we can write the equation as T+1=T+4sT + 1 = T + 4s, where TT is the initial temperature and T+1T + 1 is the temperature after the increase.
  4. Subtract and Simplify: Subtracting TT from both sides of the equation T+1=T+4sT + 1 = T + 4s gives us 1=4s1 = 4s.
  5. Find the Value: To find the value of ss, we divide both sides of the equation 1=4s1 = 4s by 44, which gives us s=14s = \frac{1}{4} or s=0.25s = 0.25 seconds.

More problems from Ratio and Quadratic equation

Question
Solve by completing the square.\newlinem2−10m−29=0m^2 - 10m - 29 = 0\newlineWrite your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.\newline`m` = ____ or `m` = _____
Get tutor helpright-arrow

Posted 1 month ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 5 5 units up of f(x)=x2 f(x)=x^2 .\newlineWrite your answer in the form a(x–h)2+k a(x–h)^2+k , where a a , h h , and k k are integers.\newlineg(x)= g(x)= ____
Get tutor helpright-arrow

Posted 1 month ago

Question
What is the range of this quadratic function?\newliney=x2−4x+4y = x^2 - 4x + 4\newlineChoices:\newline{y∣y≥2}\left\{y \mid y \geq 2\right\}\newline{y∣y≤0}\left\{y \mid y \leq 0\right\}\newline{y∣y≥0}\left\{y \mid y \geq 0\right\}\newlineall real numbers\text{all real numbers}
Get tutor helpright-arrow

Posted 1 month ago

Question
Write the equation of the parabola that passes through the points (1,0)(1,0), (2,0)(2,0), and (3,–16)(3,\text{–}16). Write your answer in the form y=a(x–p)(x–q)y = a(x – p)(x – q), where aa, pp, and qq are integers, decimals, or simplified fractions.\newline______
Get tutor helpright-arrow

Posted 1 month ago

Question
Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.\newlinef2+8f+_____f^2 + 8f + \_\_\_\_\_
Get tutor helpright-arrow

Posted 1 month ago

Question
Solve for h h .\newlineh2+39h=0 h^2 + 39h = 0 \newline\newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.\newlineh= h = ____\newline
Get tutor helpright-arrow

Posted 1 month ago

Question
Write a quadratic function with zeros −9-9 and −7-7.\newlineWrite your answer using the variable xx and in standard form with a leading coefficient of 11.\newlinef(x)=_____f(x) = \_\_\_\_\_
Get tutor helpright-arrow

Posted 2 months ago

Question
Find the equation of the axis of symmetry for the parabola y=x2y = x^2. \newlineSimplify any numbers and write them as proper fractions, improper fractions, or integers.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 88 units up of f(x)=x2f(x) = x^2.\newlineWrite your answer in the form a(x–h)2+ka(x – h)^2 + k, where aa, hh, and kk are integers.\newlineg(x)=g(x) = ______\newline
Get tutor helpright-arrow

Posted 2 months ago

Question
Solve for xx.\newlinex2=1x^2 = 1\newline\newlineWrite your answer in simplified, rationalized form.\newlinex=x = ______ or x=x = ______\newline
Get tutor helpright-arrow

Posted 2 months ago

View all (42)