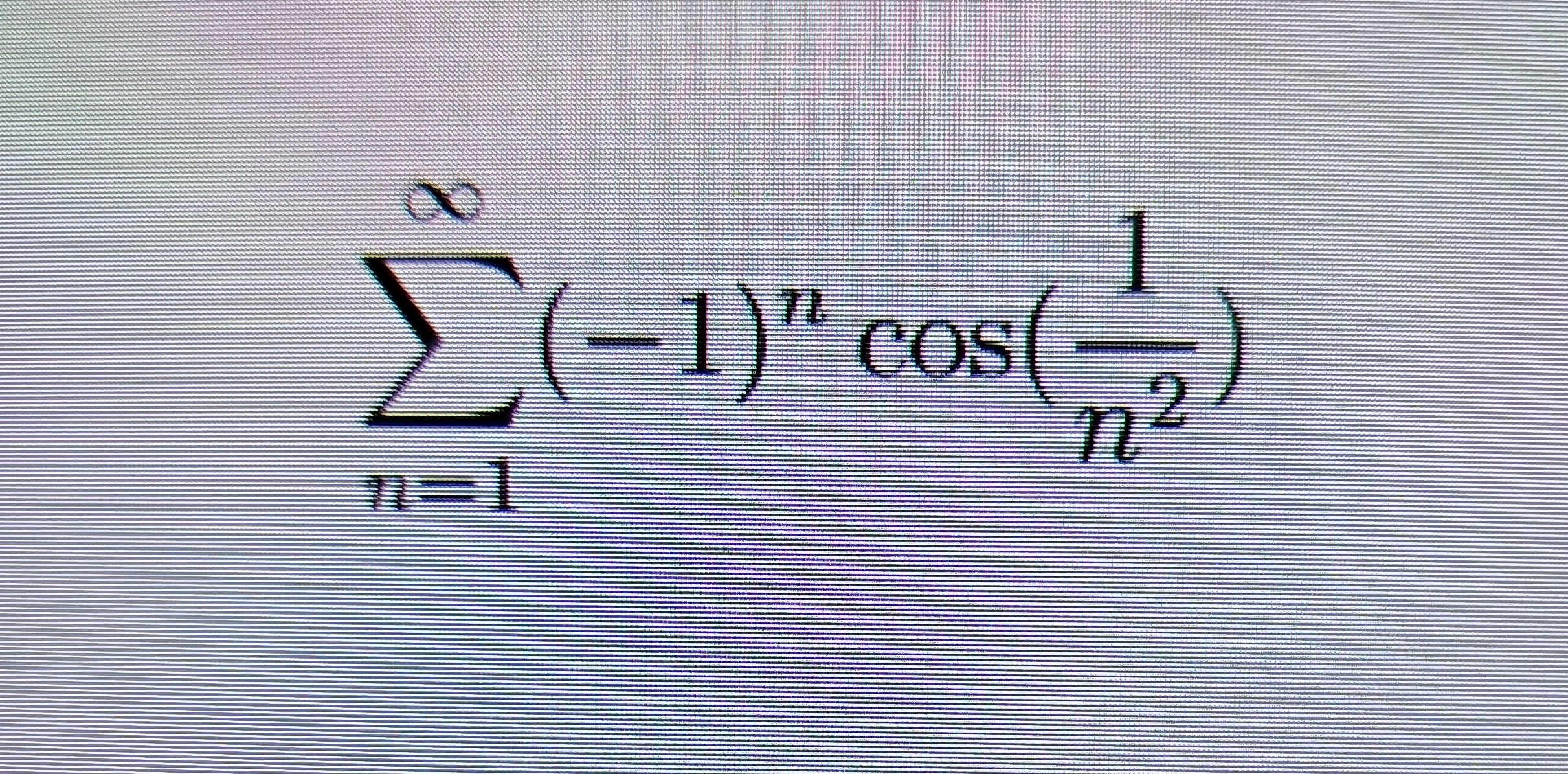AI tutor
Welcome to Bytelearn!
Let’s check out your problem:
Evaluate
Full solution
Q. Evaluate
- Check Convergence Criteria: The series given is an alternating series, where the general term is . To evaluate the sum of an infinite alternating series, we need to check if the series is convergent. This requires that the absolute value of the terms tends to zero as approaches infinity, and that the terms are decreasing in absolute value. Let's check the limit of the absolute value of the terms.
- Calculate Limit of Absolute Value: We calculate the limit of the absolute value of the terms as approaches infinity: Since the cosine function is bounded between and , and approaches as approaches infinity, we have: However, since we are considering the absolute value of the cosine function, which is always positive or zero, and the fact that is decreasing to , the terms are indeed decreasing in absolute value.
- Verify Terms Tend to Zero: Now we need to check if the terms tend to zero as approaches infinity. We already know that , but we need to consider the factor as well. Since oscillates between and , it does not affect the limit of the terms going to zero. Therefore, we can conclude that:.This means the terms of the series tend to zero as approaches infinity.
- Apply Alternating Series Test: Since the terms of the series are decreasing in absolute value and tend to zero, the series is convergent by the Alternating Series Test. However, finding the exact sum of an infinite alternating series can be challenging, especially when the terms involve a trigonometric function like cosine. In this case, the series does not correspond to a well-known sum, and it may not have a simple closed-form expression. Therefore, the sum of the series would typically be approximated numerically or left in the form of the series itself.
