AI tutor
Full solution
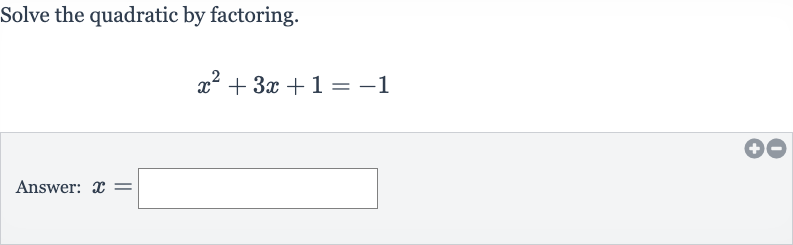
Q. Solve the quadratic by factoring.Answer:
- Set Quadratic Equation Equal: First, we need to set the quadratic equation equal to zero by adding to both sides of the equation.
- Find Two Numbers: Now, we need to find two numbers that multiply to give the constant term and add to give the coefficient of the term . The numbers that satisfy these conditions are and because: + = \)
- Factor Quadratic Equation: We can now factor the quadratic equation using these two numbers.
- Find Solutions: To find the solutions, we set each factor equal to zero and solve for .First factor: Second factor: