Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve the quadratic by factoring. 6x^(2)-18 x+3=x Answer: x=

Solve the quadratic by factoring.\newline6x2−18x+3=x 6 x^{2}-18 x+3=x \newlineAnswer: x= x=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Factor quadratics with other leading coefficientsright chevron icon

Full solution

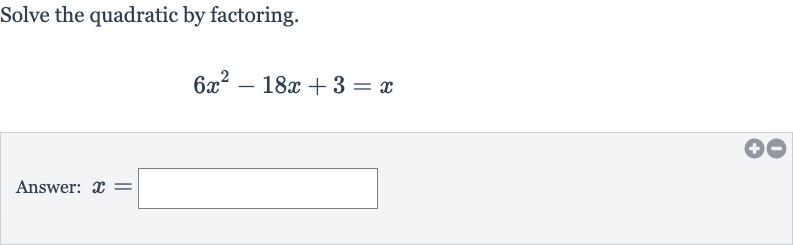
Q. Solve the quadratic by factoring.\newline6x2−18x+3=x 6 x^{2}-18 x+3=x \newlineAnswer: x= x=
  1. Move Terms to One Side: Move all terms to one side of the equation to set the quadratic equation to zero.\newlineSubtract xx from both sides to get 6x2−18x+3−x=06x^2 - 18x + 3 - x = 0.\newlineCombine like terms to get 6x2−19x+3=06x^2 - 19x + 3 = 0.
  2. Factor Quadratic Equation: Factor the quadratic equation 6x2−19x+36x^2 - 19x + 3. We need to find two numbers that multiply to (6×3)=18(6 \times 3) = 18 and add up to −19-19. The numbers that satisfy these conditions are −18-18 and −1-1 because −18×−1=18-18 \times -1 = 18 and −18+−1=−19-18 + -1 = -19.
  3. Rewrite Middle Term: Rewrite the middle term −19x-19x using the two numbers found in Step 22.\newline6x2−19x+3=6x2−18x−x+36x^2 - 19x + 3 = 6x^2 - 18x - x + 3.
  4. Factor by Grouping: Factor by grouping.\newlineGroup the first two terms and the last two terms separately.\newline(6x2−18x)−(x−3)(6x^2 - 18x) - (x - 3).\newlineFactor out the common factor of 6x6x from the first group and 11 from the second group.\newline6x(x−3)−1(x−3)6x(x - 3) - 1(x - 3).
  5. Factor Out Common Factor: Factor out the common binomial factor (x−3)(x - 3).(6x−1)(x−3)=0(6x - 1)(x - 3) = 0.
  6. Set Factors Equal: Set each factor equal to zero and solve for xx.6x−1=06x - 1 = 0 and x−3=0x - 3 = 0.For 6x−1=06x - 1 = 0, add 11 to both sides to get 6x=16x = 1, then divide by 66 to get x=16x = \frac{1}{6}.For x−3=0x - 3 = 0, add 33 to both sides to get 6x−1=06x - 1 = 000.

More problems from Factor quadratics with other leading coefficients

Question
Find the greatest common factor.\newline9v3,6v39v^3, 6v^3\newline\newlineWrite your answer as a constant times a product of single variables raised to exponents.\newline‾\underline{\hspace{1cm}}
Get tutor helpright-arrow

Posted 2 months ago

Question
Factor out the greatest common factor. If the greatest common factor is 11, just retype the polynomial.\newline6x3+9x26x^3 + 9x^2\newline______
Get tutor helpright-arrow

Posted 3 months ago

Question
Factor.\newlinek2+9k+18k^2 + 9k + 18\newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Factor.\newline9j2−169j^2 - 16\newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Factor.\newlinej3+2j2+5j+10j^3 + 2j^2 + 5j + 10
Get tutor helpright-arrow

Posted 3 months ago

Question
Factor x4+6x2+9x^4 + 6x^2 + 9 completely.\newlineAll factors in your answer should have integer coefficients.\newline______
Get tutor helpright-arrow

Posted 3 months ago

Question
Factor.\newlinez2+13z+22z^2 + 13z + 22\newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
Simplify the expression: \newline5x+9x−4x−2x5x + 9x - 4x - 2x \newline______
Get tutor helpright-arrow

Posted 4 months ago

Question
3−323 - 3^2
Get tutor helpright-arrow

Posted 4 months ago

Question
5−525 - 5^2
Get tutor helpright-arrow

Posted 2 months ago

View all (143)