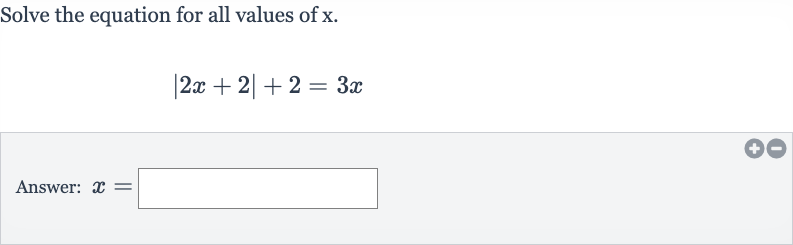Solve the equation for all values of x. |2x+2|+2=3x Answer: x=

Question

Solve the equation for all values of  x.  |2x+2|+2=3x Answer:  x=

Bytelearn · Accepted Answer

Isolate absolute value: We have the equation |2x+2|+2=3x. To solve for x, we first need to isolate the absolute value expression on one side of the equation. Subtract 2 from both sides of the equation to isolate the absolute value. |2x+2| + 2 - 2 = 3x - 2 |2x+2| = 3x - 2Split into two equations: Now we have |2x+2| = 3x - 2. The absolute value equation can be split into two separate equations, because the expression inside the absolute value can be either positive or negative. The two cases are: 1. 2x + 2 = 3x - 2 when 2x + 2 is positive or zero. 2. -(2x + 2) = 3x - 2 when 2x + 2 is negative.Solve first case: Let's solve the first case: 2x + 2 = 3x - 2. Subtract 2x from both sides to get x on one side: 2x + 2 - 2x = 3x - 2 - 2x 2 = x - 2 Now, add 2 to both sides to solve for x: 2 + 2 = x - 2 + 2 4 = xSolve second case: Let's solve the second case: -(2x + 2) = 3x - 2. First, distribute the negative sign: -2x - 2 = 3x - 2 Now, add 2x to both sides to get x on one side: -2x - 2 + 2x = 3x - 2 + 2x -2 = 5x - 2 Next, add 2 to both sides to isolate the 5x term: -2 + 2 = 5x - 2 + 2 0 = 5x Finally, divide both sides by 5 to solve for x: 0 / 5 = 5x / 5 0 = xCheck solutions: We have found two potential solutions for x: x = 4 and x = 0. However, we must check these solutions in the original equation to ensure they do not result from extraneous solutions introduced by squaring the equation. Check x = 4 in the original equation: |2(4)+2|+2 = 3(4) |8+2|+2 = 12 |10|+2 = 12 10+2 = 12 12 = 12 which is true, so x = 4 is a valid solution. Check x = 0 in the original equation: |2(0)+2|+2 = 3(0) |0+2|+2 = 0 |2|+2 = 0 2+2 = 0 which is not true, so x = 0 is not a valid solution.

Solve the equation for all values of $x$ . $\newline$ $|2 x+2|+2=3 x$ $\newline$ Answer: $x=$

Full solution

More problems from Evaluate absolute value expressions

Related topics

Solve the equation for all values of x x x.\newline∣2x+2∣+2=3x |2 x+2|+2=3 x ∣2x+2∣+2=3x\newlineAnswer: x= x= x=

Solve the equation for all values of $x$ . $\newline$ $|2 x+2|+2=3 x$ $\newline$ Answer: $x=$