Full solution
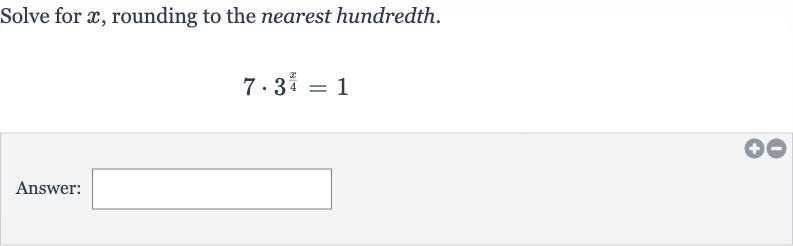
Q. Solve for , rounding to the nearest hundredth.Answer:
- Isolate exponential term: First, we need to isolate the exponential term on one side of the equation. Since we are given , we can divide both sides by to get on its own.Calculation:
- Solve for : Next, we need to solve for in the exponential equation. To do this, we can take the natural logarithm () of both sides of the equation to remove the base exponent.Calculation:
- Simplify using logarithms: Using the property of logarithms that , we can simplify the left side of the equation.Calculation:
- Multiply by : Now, we solve for by multiplying both sides of the equation by .Calculation:
- Calculate numerical value of x: We can now use a calculator to find the numerical value of .Calculation:
- Round to nearest hundredth: Finally, we round the value of to the nearest hundredth.Calculation:
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help