Full solution
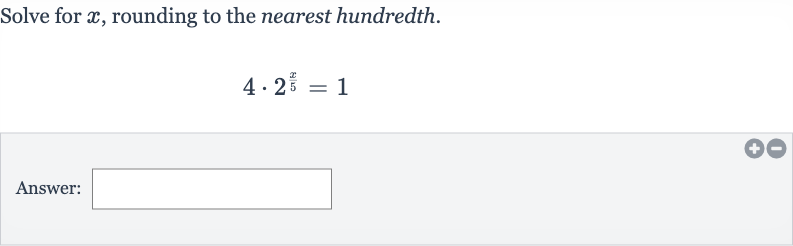
Q. Solve for , rounding to the nearest hundredth.Answer:
- Isolate exponential term: First, we need to isolate the exponential term on one side of the equation. Since we are given , we can divide both sides by to get .
- Recognize fraction as negative exponent: Next, we recognize that is because . So we can rewrite the equation as .
- Set exponents equal: Since the bases are the same and the equation is an equality, we can set the exponents equal to each other. This gives us .
- Multiply to isolate : To solve for , we multiply both sides of the equation by to isolate . This gives us .
- Final solution: Multiplying by gives us .
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help