Full solution
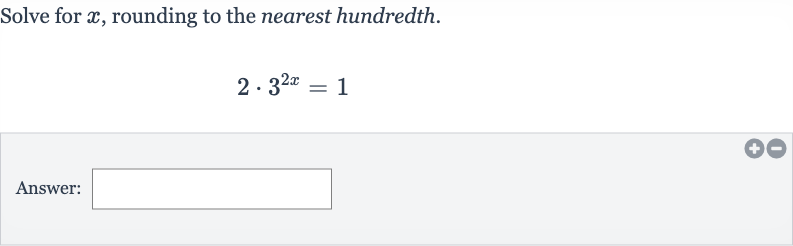
Q. Solve for , rounding to the nearest hundredth.Answer:
- Understand and Isolate Exponential Term: Understand the equation and isolate the exponential term.We have the equation . To solve for , we first need to isolate the term with the exponent. We do this by dividing both sides of the equation by .
- Take Logarithm to Solve: Take the logarithm of both sides to solve for the exponent.To solve for the exponent , we can take the natural logarithm (ln) of both sides of the equation.
- Apply Power Rule of Logarithms: Apply the power rule of logarithms. The power rule of logarithms states that . We apply this rule to the left side of the equation.
- Solve for x: Solve for x.Now we can solve for x by dividing both sides of the equation by .
- Calculate x Value: Calculate the value of x using a calculator.Using a calculator, we find:Rounded to the nearest hundredth,
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help