Full solution
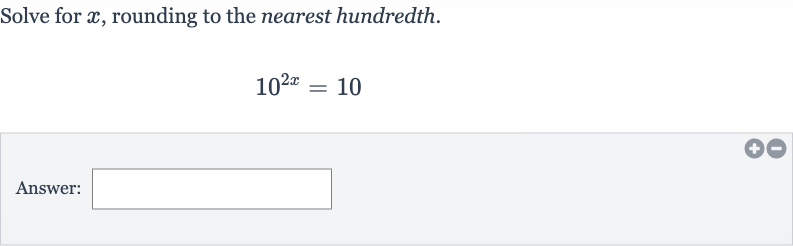
Q. Solve for , rounding to the nearest hundredth.Answer:
- Set up equation: Set up the equation based on the given problem.We have the equation . To solve for , we need to find the value of that makes the equation true.
- Use logarithm property: Use the property of logarithms to solve for . Since is the base of the exponential function, we can take the logarithm with base of both sides of the equation to isolate . This gives us:
- Apply power rule: Apply the power rule of logarithms.The power rule states that , where is the base, is the argument, and is the exponent. Applying this rule, we get:
- Simplify equation: Simplify the equation.Since is equal to (because is the base of the common logarithm), the equation simplifies to:Which further simplifies to:
- Solve for x: Solve for x.To find x, we divide both sides of the equation by :
- Convert to decimal: Convert the exact answer to a decimal rounded to the nearest hundredth.
More problems from Find trigonometric functions using a calculator
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
Question
. Find the value of in degrees.Write your answer in simplified, rationalized form. Do not round.____
Get tutor helpQuestionGet tutor help