Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Solve for the exact value of
x.

2ln(2x-2)+12=8
Answer:

Solve for the exact value of $x$ . $\newline$ $2 \ln (2 x-2)+12=8$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Find trigonometric ratios using a Pythagorean or reciprocal identity

Full solution

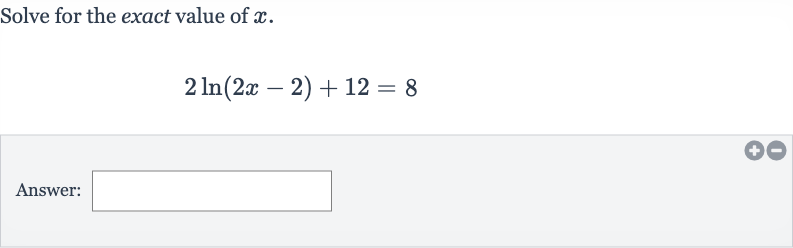
Q. Solve for the exact value of

x

.

\newline

2 \ln (2 x-2)+12=8

\newline

Answer:

Isolate logarithmic expression: Isolate the logarithmic expression. $\newline$ We start by subtracting $12$ from both sides of the equation to isolate the logarithmic term. $\newline$ $2\ln(2x-2) + 12 - 12 = 8 - 12$ $\newline$ $2\ln(2x-2) = -4$
Divide to solve ln term: Divide both sides by $2$ to solve for the ln term. $\newline$ Divide both sides by $2$ to isolate $\ln(2x-2)$ . $\newline$ $(2\ln(2x-2)) / 2 = -4 / 2$ $\newline$ $\ln(2x-2) = -2$
Exponentiate to remove ln: Exponentiate both sides to remove the natural logarithm. $\newline$ We raise $e$ to the power of both sides of the equation to remove the natural logarithm. $\newline$ $e^{\ln(2x-2)} = e^{-2}$ $\newline$ $2x - 2 = e^{-2}$
Add to solve for $2x$ : Add $2$ to both sides to solve for $2x$ . $\newline$ Add $2$ to both sides of the equation to isolate the term with $x$ . $\newline$ $2x - 2 + 2 = e^{(-2)} + 2$ $\newline$ $2x = e^{(-2)} + 2$
Divide to solve for x: Divide both sides by $2$ to solve for $x$ . $\newline$ Divide both sides by $2$ to find the value of $x$ . $\newline$ $\frac{2x}{2} = \frac{e^{(-2)} + 2}{2}$ $\newline$ $x = \frac{e^{(-2)} + 2}{2}$

More problems from Find trigonometric ratios using a Pythagorean or reciprocal identity

Question

\cos(\theta)=\frac{8}{17}

0^\circ<\theta<90^\circ

\sec(\theta)

? Write your answer in simplified, rationalized form.

\sec(\theta)=

Posted 2 months ago

Question

\cos(x) = -0.3

\sin(90^\circ - x)

Posted 2 months ago

Question

\csc(\theta) = \frac{17}{8}

0^\circ < \theta < 90^\circ

\tan(\theta)

\newline

Write your answer in simplified, rationalized form.

\newline

\tan(\theta) =

\newline

Posted 2 months ago

Question

Is the sine function even or odd?

\newline

\newline

\text{[A]even}

\newline

\text{[B]odd}

Posted 2 months ago

Question

Write the sentence as an equation.

\newline

89

is equal to the quotient of

y

17

\newline

/

) if you want to use a division sign.

\newline

Posted 1 month ago

Question

Write the sentence as an equation.

\newline

45

is equal to the quotient of

z

5

\newline

/

) if you want to use a division sign.

\newline

Posted 3 months ago

Question

h

\newline

h^2 + 24h = 0

\newline

Write each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.

\newline

h =

Posted 3 months ago

Question

h^2 - 4h = 0

Posted 1 month ago

Question

Combine the like terms to create an equivalent expression:

\newline

-k-(-8k)=\square

Posted 4 months ago

Question

Which of the following degree measures is equal to

5 \pi

\newline

(The number of degrees of arc in a circle is

360

. The number of radians of arc in a circle is

2 \pi

\newline

1

\newline

144^{\circ}

\newline

900^{\circ}

\newline

1,080^{\circ}

\newline

1,800^{\circ}

Posted 1 month ago