AI tutor
Full solution
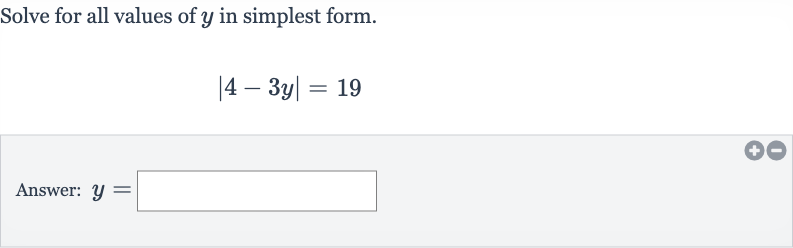
Q. Solve for all values of in simplest form.Answer:
- Absolute Value Equation Explanation: We are given the absolute value equation . The absolute value of a number is the distance of that number from zero on the number line, which means it is always non-negative. Therefore, the expression inside the absolute value can be either positive or equal to , or negative and its absolute value equal to . We can set up two separate equations to solve for : or
- First Equation Solution: Let's solve the first equation . Subtract from both sides to isolate the term with . Now, divide both sides by to solve for .
- Second Equation Solution: Now let's solve the second equation .Subtract from both sides to isolate the term with .Now, divide both sides by to solve for . or
More problems from Evaluate absolute value expressions
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help