AI tutor
Full solution
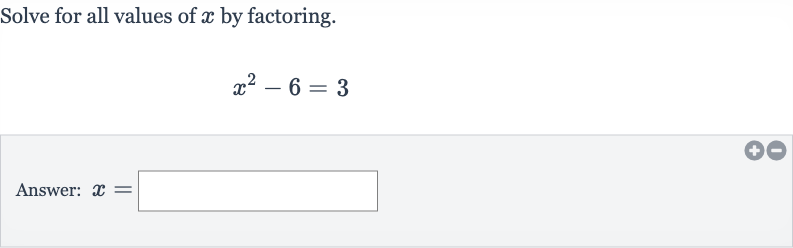
Q. Solve for all values of by factoring.Answer:
- Move to Standard Form: Write the equation in standard form by moving all terms to one side.We start with the equation and move the constant term on the right side to the left side to get .Now the equation is .
- Factor Quadratic Equation: Factor the quadratic equation.We need to factor . This is a difference of squares, which can be factored into .
- Apply Zero Product Property: Solve for using the zero product property.The zero product property states that if a product of two factors is zero, then at least one of the factors must be zero. Therefore, we set each factor equal to zero and solve for . or .
- Solve for x: Solve each equation for x.For , we subtract from both sides to get .For , we add to both sides to get .