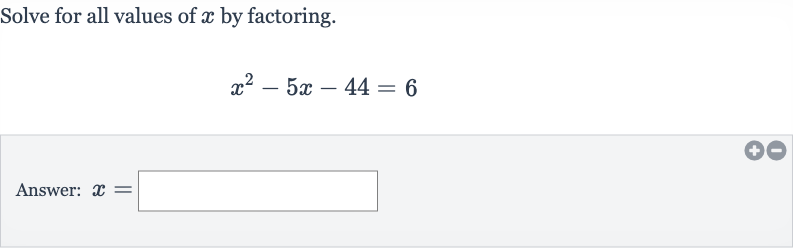
Solve for all values of x by factoring. x^(2)-5x-44=6 Answer: x=

Question

Solve for all values of  x by factoring.  x^(2)-5x-44=6 Answer:  x=

Bytelearn · Accepted Answer

Bring to one side: Bring all terms to one side of the equation to set it equal to zero. x^2 - 5x - 44 = 6 Subtract 6 from both sides to get: x^2 - 5x - 50 = 0Identify coefficients and constant: Identify the coefficients and constant term in the quadratic equation. The quadratic equation is now in the form ax^2 + bx + c = 0, where: a = 1 (coefficient of x^2) b = -5 (coefficient of x) c = -50 (constant term)Find two numbers: Find two numbers that multiply to give ac (a*c) and add to give b. In this case, ac = 1*(-50) = -50 and b = -5. The two numbers that multiply to -50 and add to -5 are -10 and 5. -10 * 5 = -50 -10 + 5 = -5Rewrite with two numbers: Rewrite the equation using the two numbers found in Step 3 to split the middle term. x^2 - 10x + 5x - 50 = 0 Group the terms: (x^2 - 10x) + (5x - 50) = 0Factor by grouping: Factor by grouping. Factor out the greatest common factor from each group. x(x - 10) + 5(x - 10) = 0 Now factor out the common binomial factor (x - 10): (x - 10)(x + 5) = 0Set equal and solve: Set each factor equal to zero and solve for x. x - 10 = 0 or x + 5 = 0 Solve each equation: x = 10 or x = -5

Solve for all values of $x$ by factoring. $\newline$ $x^{2}-5 x-44=6$ $\newline$ Answer: $x=$

Full solution

More problems from Factor quadratics with leading coefficient 1

Related topics

Solve for all values of x x x by factoring.\newlinex2−5x−44=6 x^{2}-5 x-44=6 x2−5x−44=6\newlineAnswer: x= x= x=

Solve for all values of $x$ by factoring. $\newline$ $x^{2}-5 x-44=6$ $\newline$ Answer: $x=$