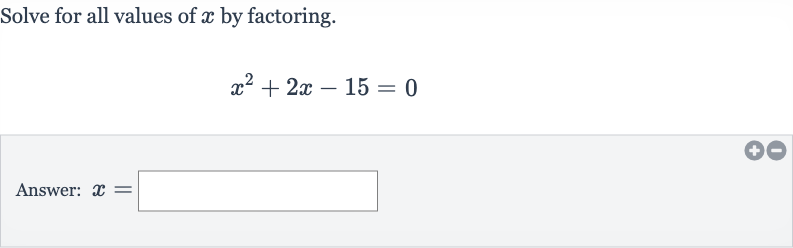
Solve for all values of x by factoring. x^(2)+2x-15=0 Answer: x=

Question

Solve for all values of  x by factoring.  x^(2)+2x-15=0 Answer:  x=

Bytelearn · Accepted Answer

Identify Equation: Identify the quadratic equation to be factored. We have the quadratic equation x^2 + 2x - 15 = 0. We need to find two numbers that multiply to give -15 (the constant term) and add up to 2 (the coefficient of the x term).Find Numbers: Find two numbers that multiply to -15 and add up to 2. The numbers 5 and -3 satisfy these conditions because 5 * (-3) = -15 and 5 + (-3) = 2.Rewrite Equation: Rewrite the quadratic equation using the two numbers found. The equation x^2 + 2x - 15 = 0 can be rewritten as x^2 + 5x - 3x - 15 = 0 by splitting the middle term using the numbers 5 and -3.Factor by Grouping: Factor by grouping. Group the terms to factor by common terms: (x^2 + 5x) - (3x + 15) = 0. Factor out an x from the first group and a -3 from the second group: x(x + 5) - 3(x + 5) = 0.Factor Common Factor: Factor out the common binomial factor. Since both groups contain the factor (x + 5), factor it out: (x - 3)(x + 5) = 0.Solve for x: Solve for x by setting each factor equal to zero. Set the first factor equal to zero: x - 3 = 0, which gives x = 3. Set the second factor equal to zero: x + 5 = 0, which gives x = -5.

Solve for all values of $x$ by factoring. $\newline$ $x^{2}+2 x-15=0$ $\newline$ Answer: $x=$

Full solution

More problems from Factor quadratics with leading coefficient 1

Related topics

Solve for all values of x x x by factoring.\newlinex2+2x−15=0 x^{2}+2 x-15=0 x2+2x−15=0\newlineAnswer: x= x= x=

Solve for all values of $x$ by factoring. $\newline$ $x^{2}+2 x-15=0$ $\newline$ Answer: $x=$