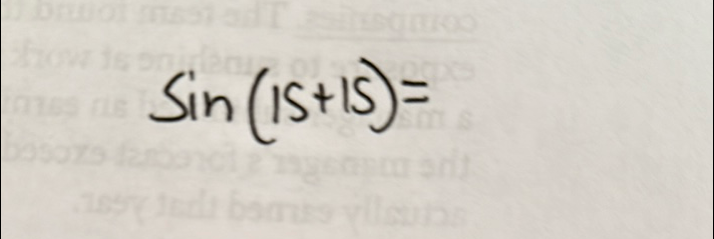Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

sin(15+15)=

sin⁡(15+15)= \sin (15+15)=

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Grade 8right chevron icon
Multiply using the distributive propertyright chevron icon

Full solution

Q. sin⁡(15+15)= \sin (15+15)=
  1. Recognize Identity: Recognize that the expression sin⁡(15+15)\sin(15+15) can be rewritten using the angle sum identity for sine. The angle sum identity for sine is sin⁡(A+B)=sin⁡(A)cos⁡(B)+cos⁡(A)sin⁡(B)\sin(A + B) = \sin(A)\cos(B) + \cos(A)\sin(B). Here, AA and BB are both 1515 degrees, so we can apply the identity.
  2. Apply Identity: Apply the angle sum identity for sine to the expression sin⁡(15+15)\sin(15+15).sin⁡(15+15)=sin⁡(15)cos⁡(15)+cos⁡(15)sin⁡(15)\sin(15+15) = \sin(15)\cos(15) + \cos(15)\sin(15)
  3. Calculate Values: Recognize that sin⁡(15)\sin(15) and cos⁡(15)\cos(15) need to be calculated or looked up, as they are not standard angles. However, we know that sin⁡(30)\sin(30) is a standard angle and its value is 12\frac{1}{2}. Since 15+1515+15 equals 3030, we can simplify the expression to sin⁡(30)\sin(30).
  4. Find Expression Value: Use the known value of sin⁡(30)\sin(30) to find the value of the expression.sin⁡(30)=12\sin(30) = \frac{1}{2}

More problems from Multiply using the distributive property

Question
Simplify the expression:\newline7z2+3z27z^2 + 3z^2 \newline ______
Get tutor helpright-arrow

Posted 1 month ago

Question
Simplify the expression:\newline10f+8f−9f−6f10f + 8f - 9f - 6f\newline____
Get tutor helpright-arrow

Posted 1 month ago

Question
Subtract.\newline(3h+6)−(3h+2)(3h + 6) - (3h + 2)\newline____
Get tutor helpright-arrow

Posted 1 month ago

Question
Simplify the expression:\newline2p−p2p - p \newline______
Get tutor helpright-arrow

Posted 2 months ago

Question
A school charges $6\$6 per ticket to a dance. They spend $300\$300 on music and decorations. The expression 6n−3006n-300 describes the amount of profit the school makes from the dance if nn students buy tickets. What does 6n6n represent in this context?\newlineChoices:\newline(A)The amount of money the school charges for all `\n` tickets\newline(B)The amount of money the school spends on the dance\newline(C)The number of students who buy tickets\newline(D)The profit the school earns per ticket
Get tutor helpright-arrow

Posted 2 months ago

Question
Which is equal to cos⁡60∘ \cos 60^\circ ? \newlineChoices:\newline(A) sin⁡30∘ \sin 30^\circ \newline(B) sin⁡60∘ \sin 60^\circ \newline(C) cos⁡30∘ \cos 30^\circ \newline(D) cos⁡60∘ \cos 60^\circ
Get tutor helpright-arrow

Posted 3 months ago

Question
Which is equal to sin⁡45∘\sin 45^\circ? \newlineChoices:\newline(A) cos⁡45∘\cos 45^\circ\newline(B) cos⁡55∘\cos 55^\circ\newline(C) sin⁡55∘\sin 55^\circ\newline(D) sin⁡35∘\sin 35^\circ
Get tutor helpright-arrow

Posted 1 month ago

Question
Which is equal to cos⁡75∘\cos 75^\circ? \newlineChoices:\newline(A) sin⁡15∘\sin 15^\circ\newline(B) sin⁡75∘\sin 75^\circ\newline(C) cos⁡15∘\cos 15^\circ\newline(D) cos⁡25∘\cos 25^\circ
Get tutor helpright-arrow

Posted 1 month ago

Question
Which is equal to sin⁡30∘ \sin 30^\circ ? \newlineChoices:\newline(A) cos⁡60∘ \cos 60^\circ \newline(B) cos⁡30∘ \cos 30^\circ \newline(C) sin⁡60∘ \sin 60^\circ \newline(D) sin⁡30∘ \sin 30^\circ
Get tutor helpright-arrow

Posted 1 month ago

Question
Which is equal to cos⁡45∘ \cos 45^\circ ? \newlineChoices:\newline(A) sin⁡45∘ \sin 45^\circ \newline(B) sin⁡55∘ \sin 55^\circ \newline(C) cos⁡55∘ \cos 55^\circ \newline(D) cos⁡35∘ \cos 35^\circ
Get tutor helpright-arrow

Posted 1 month ago

View all (30)