Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Simplify to a single power of 3 :

3^(5)×3^(2)
Answer: 3^◻

Simplify to a single power of $3$ : $\newline$ $3^{5} \times 3^{2}$ $\newline$ Answer: $3 ^\square$

View step-by-step help

View step-by-step help

Multiply fractions

Full solution

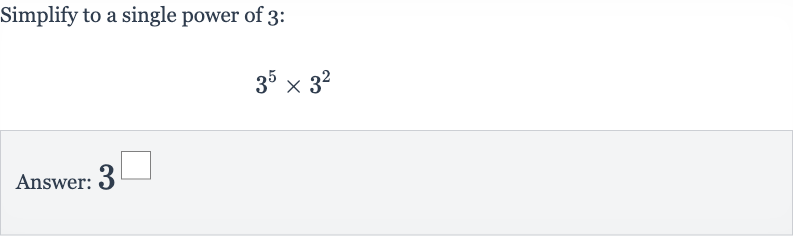
Q. Simplify to a single power of

3

:

\newline

3^{5} \times 3^{2}

\newline

Answer:

3 ^\square

Apply Exponent Property: To simplify the expression $3^{5}\times3^{2}$ to a single power of $3$ , we use the property of exponents that states when multiplying like bases, we add the exponents. $\newline$ Calculation: $3^{5}\times3^{2} = 3^{5+2}$
Add Exponents: Now we add the exponents $5$ and $2$ . $\newline$ Calculation: $5 + 2 = 7$
Simplify Expression: After adding the exponents, we get the simplified expression as $3^{7}$ .

More problems from Multiply fractions

Question

\newline

2x-2=0

Posted 3 months ago

Question

2x+3 = 2

\newline

x

Posted 3 months ago

Question

2+8

Posted 3 months ago

Question

6+12

Posted 3 months ago

Question

\newline

(\frac{3}{5})\times(\frac{1}{6})=

\newline

\newline

Posted 3 months ago

Question

2x+3 = 2

\newline

x.

Posted 3 months ago

Question

Simplify to a single power of

6

\newline

6^{3} \cdot 6^{2}

\newline

6 ^\square

Posted 3 months ago

Question

z

makes the equation below true?

\newline

9 z-2=79

\newline

3

\newline

9

\newline

13

\newline

79

Posted 2 months ago

Question

y

makes the equation below true?

\newline

5 y+2=17

\newline

2

\newline

3

\newline

6

\newline

17

Posted 2 months ago

Question

\begin{array}{l}y+6=5(x-4) \\ y=\square\end{array}

Posted 4 hours ago