Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Simplify the following expression to simplest form using only positive exponents. (64x^(-24)y^(12))^((4)/(3)) Answer:

Simplify the following expression to simplest form using only positive exponents.\newline(64x−24y12)43 \left(64 x^{-24} y^{12}\right)^{\frac{4}{3}} \newlineAnswer:\newline

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 1right chevron icon
Multiplication with rational exponentsright chevron icon

Full solution

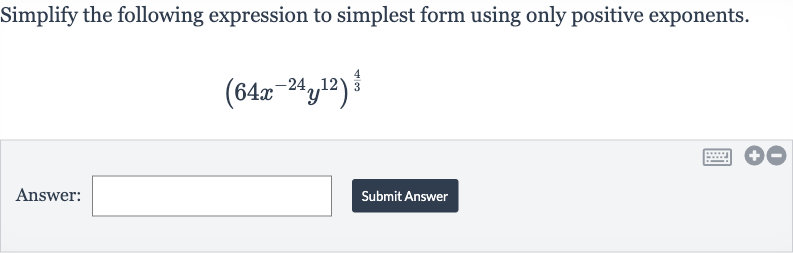
Q. Simplify the following expression to simplest form using only positive exponents.\newline(64x−24y12)43 \left(64 x^{-24} y^{12}\right)^{\frac{4}{3}} \newlineAnswer:\newline
  1. Apply Power to Factors: Apply the power to each factor inside the parentheses.\newlineWe have the expression (64x(−24)y(12))(43)(64x^{(-24)}y^{(12)})^{(\frac{4}{3})}. To simplify, we will apply the exponent (43)(\frac{4}{3}) to each factor inside the parentheses separately.
  2. Simplify Base 6464: Simplify the numerical base 6464 raised to the power of (4/3)(4/3). 6464 is 44 raised to the power of 33 (434^3), so we can rewrite 6464 as 434^3 and then apply the exponent (4/3)(4/3) to it. (43)(4)/(3)=43∗(4/3)=44=256(4^3)^{(4)/(3)} = 4^{3*(4/3)} = 4^4 = 256
  3. Simplify Variable xx: Simplify the variable xx raised to the power of (−24)(-24) and then to the power of (4/3)(4/3). We apply the exponent (4/3)(4/3) to x(−24)x^{(-24)} by multiplying the exponents. (x(−24))(4/3)=x((−24)∗(4/3))=x(−32)(x^{(-24)})^{(4/3)} = x^{((-24)*(4/3))} = x^{(-32)} Since we want only positive exponents, we can write x(−32)x^{(-32)} as 1/x321/x^{32}.
  4. Simplify Variable yy: Simplify the variable yy raised to the power of 1212 and then to the power of (4/3)(4/3). We apply the exponent (4/3)(4/3) to y12y^{12} by multiplying the exponents. (y12)(4/3)=y12∗(4/3)=y16(y^{12})^{(4/3)} = y^{12*(4/3)} = y^{16}
  5. Combine Results: Combine the results from Steps 22, 33, and 44.\newlineWe now combine the simplified terms to get the final expression.\newline256×(1/x32)×y16256 \times (1/x^{32}) \times y^{16}
  6. Final Expression: Write the final expression in simplest form.\newlineThe final expression is already in simplest form with only positive exponents.\newline256⋅y16/x32256 \cdot y^{16} / x^{32}

More problems from Multiplication with rational exponents

Question
Simplify.\newline811281^{\frac{1}{2}}\newline_____________
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify.\newline(−32)1/5(-32)^{1/5}\newline______
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify. Assume all variables are positive.\newlinew43w73\frac{w^{\frac{4}{3}}}{w^{\frac{7}{3}}}\newlineWrite your answer in the form AA or AB\frac{A}{B}, where AA and BB are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Simplify. Assume all variables are positive.\newline(2r13)8(2r^{\frac{1}{3}})^8\newline\newlineWrite your answer in the form AA or AB\frac{A}{B}, where AA and BB are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Simplify. Assume all variables are positive.\newlinev74⋅v94v^{\frac{7}{4}} \cdot v^{\frac{9}{4}}\newline\newlineWrite your answer in the form AA or AB\frac{A}{B}, where AA and BB are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.\newline______\newline
Get tutor helpright-arrow

Posted 5 months ago

Question
Simplify.\newline114⋅1141^{\frac{1}{4}} \cdot 1^{\frac{1}{4}}\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Simplify. \newline32(−1/5)32^{(-1/5)}
Get tutor helpright-arrow

Posted 7 months ago

Question
Simplify. Assume all variables are positive.\newlinew32w52\frac{w^{\frac{3}{2}}}{w^{\frac{5}{2}}}\newline\newlineWrite your answer in the form AA or AB\frac{A}{B}, where AA and BB are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.\newline______\newline
Get tutor helpright-arrow

Posted 9 months ago

Question
Simplify. Assume all variables are positive.\newline(27x)23(27x)^{\frac{2}{3}}\newline\newlineWrite your answer in the form AA or AB\frac{A}{B}, where AA and BB are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.\newline______
Get tutor helpright-arrow

Posted 8 months ago

Question
Simplify. Assume all variables are positive.\newlinex14x114\frac{x^{\frac{1}{4}}}{x^{\frac{11}{4}}}\newline\newlineWrite your answer in the form AA or AB\frac{A}{B}, where AA and BB are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.\newline______\newline
Get tutor helpright-arrow

Posted 7 months ago

View all (744)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant