Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Simplify the following expression to simplest form using only positive exponents.

(32x^(-20)y^(-5))^(-(2)/(5))
Answer:

Simplify the following expression to simplest form using only positive exponents. $\newline$ $\left(32 x^{-20} y^{-5}\right)^{-\frac{2}{5}}$ $\newline$ Answer:

View step-by-step help

View step-by-step help

Multiplication with rational exponents

Full solution

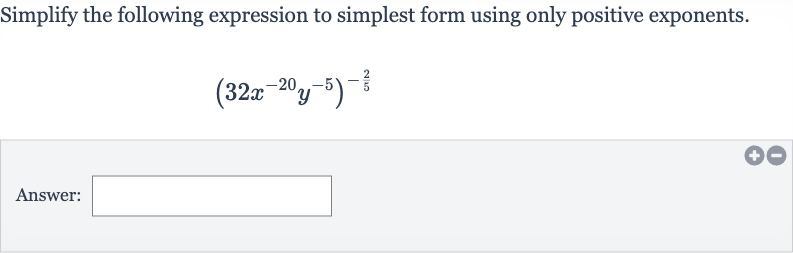
Q. Simplify the following expression to simplest form using only positive exponents.

\newline

\left(32 x^{-20} y^{-5}\right)^{-\frac{2}{5}}

\newline

Answer:

Apply negative exponent rule: Apply the negative exponent rule to the entire expression. $\newline$ The negative exponent rule states that $a^{-n} = \frac{1}{a^n}$ . We will apply this rule to the expression $(32x^{-20}y^{-5})^{-\frac{2}{5}}$ .
Distribute exponent to each factor: Distribute the exponent to each factor inside the parentheses. $\newline$ When raising a power to a power, you multiply the exponents. In this case, we have a product inside the parentheses, so we will distribute the exponent to each factor. $\newline$ $(32x^{(-20)}y^{(-5)})^{(-(2)/(5))} = 32^{(-(2)/(5))} \times x^{(-20 \times (-(2)/(5)))} \times y^{(-5 \times (-(2)/(5)))}$
Simplify each factor separately: Simplify each factor separately. $\newline$ Now we simplify each factor by applying the exponent to each base. $\newline$ $32^{-(2)/(5)} = \frac{1}{32^{2/5}}$ $\newline$ $x^{(-20 \cdot (-(2)/(5)))} = x^{20 \cdot (2/5)} = x^{8}$ $\newline$ $y^{(-5 \cdot (-(2)/(5)))} = y^{5 \cdot (2/5)} = y^{2}$
Calculate fifth root and square: Calculate the fifth root of $32$ and then square it. $\newline$ $32^{(2/5)}$ means the fifth root of $32$ squared. The fifth root of $32$ is $2$ , because $2^5 = 32$ . $\newline$ $(2^5)^{(2/5)} = 2^{(5*(2/5))} = 2^2 = 4$
Combine simplified factors: Combine the simplified factors. $\newline$ Now we combine the simplified factors to get the final expression with positive exponents. $\newline$ $\frac{1}{32^{\frac{2}{5}}} \times x^{8} \times y^{2} = \frac{1}{4} \times x^{8} \times y^{2}$
Write final simplified expression: Write the final simplified expression. $\newline$ The final expression is: $\newline$ $\frac{1}{4x^8y^2}$

More problems from Multiplication with rational exponents

Question

\newline

81^{\frac{1}{2}}

\newline

Posted 9 months ago

Question

\newline

(-32)^{1/5}

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{4}{3}}}{w^{\frac{7}{3}}}

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

(2r^{\frac{1}{3}})^8

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 5 months ago

Question

Simplify. Assume all variables are positive.

\newline

v^{\frac{7}{4}} \cdot v^{\frac{9}{4}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 5 months ago

Question

\newline

1^{\frac{1}{4}} \cdot 1^{\frac{1}{4}}

\newline

Posted 5 months ago

Question

\newline

32^{(-1/5)}

Posted 7 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{w^{\frac{3}{2}}}{w^{\frac{5}{2}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 9 months ago

Question

Simplify. Assume all variables are positive.

\newline

(27x)^{\frac{2}{3}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

Posted 8 months ago

Question

Simplify. Assume all variables are positive.

\newline

\frac{x^{\frac{1}{4}}}{x^{\frac{11}{4}}}

\newline

\newline

Write your answer in the form

A

\frac{A}{B}

A

B

are constants or variable expressions that have no variables in common. All exponents in your answer should be positive.

\newline

\newline

Posted 7 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant