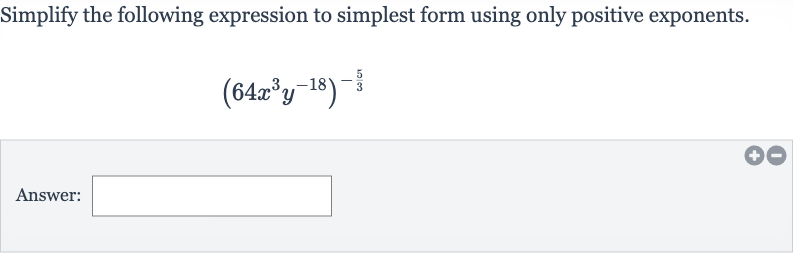
Simplify the following expression to simplest form using only positive exponents. (64x^(3)y^(-18))^(-(5)/(3)) Answer:

Question

Simplify the following expression to simplest form using only positive exponents.  (64x^(3)y^(-18))^(-(5)/(3)) Answer:

Bytelearn · Accepted Answer

Apply Negative Exponent Rule: Apply the negative exponent rule to the entire expression. The negative exponent rule states that a^(-n) = 1/(a^n). We will apply this rule to the expression (64x^(3)y^(-18))^(-(5)/(3)).Distribute Exponent to Factors: Distribute the exponent to each factor inside the parentheses. When raising a power to a power, you multiply the exponents. Here, we have a product inside the parentheses, so we distribute the exponent to each factor. (64x^(3)y^(-18))^(-(5)/(3)) = 64^(-(5)/(3)) * x^(3*(-(5)/(3))) * y^(-18*(-(5)/(3)))Simplify Each Factor: Simplify each factor separately. Now we simplify each factor by multiplying the exponents. 64^(-(5)/(3)) = 1/(64^(5/3)) x^(3*(-(5)/(3))) = x^(-5) y^(-18*(-(5)/(3))) = y^(30)Simplify 64^(5/3) Term: Simplify the 64^(5/3) term. 64 is 4^3, so we can rewrite 64^(5/3) as (4^3)^(5/3). When raising a power to a power, we multiply the exponents. (4^3)^(5/3) = 4^(3*(5/3)) = 4^5Calculate 4^5: Calculate 4^5. 4^5 = 4 * 4 * 4 * 4 * 4 = 1024Substitute 4^5: Substitute 4^5 back into the expression. Now we substitute 1024 for 4^5 in the expression from Step 3. 1/(64^(5/3)) = 1/1024Combine Simplified Factors: Combine all the simplified factors. Now we combine all the simplified factors to get the final expression with positive exponents. 1/(1024) * x^(-5) * y^(30)Rewrite x^(-5): Rewrite x^(-5) with a positive exponent. To write x^(-5) with a positive exponent, we use the rule a^(-n) = 1/(a^n). x^(-5) = 1/(x^5)Combine into Single Fraction: Combine all terms into a single fraction. Now we combine all terms into a single fraction to get the final answer. 1/(1024 * x^5) * y^(30)

Simplify the following expression to simplest form using only positive exponents. $\newline$ $\left(64 x^{3} y^{-18}\right)^{-\frac{5}{3}}$ $\newline$ Answer:

Full solution

More problems from Multiplication with rational exponents

Related topics

Simplify the following expression to simplest form using only positive exponents.\newline(64x3y−18)−53 \left(64 x^{3} y^{-18}\right)^{-\frac{5}{3}} (64x3y−18)−35​\newlineAnswer:

Simplify the following expression to simplest form using only positive exponents. $\newline$ $\left(64 x^{3} y^{-18}\right)^{-\frac{5}{3}}$ $\newline$ Answer: