Simplify the following expression to simplest form using only positive exponents. $\newline$ $\left(100 x^{-4} y^{2}\right)^{-\frac{5}{2}}$ $\newline$ Answer:

View step-by-step help

Home

Math Problems

Algebra 1

Multiplication with rational exponents

Full solution

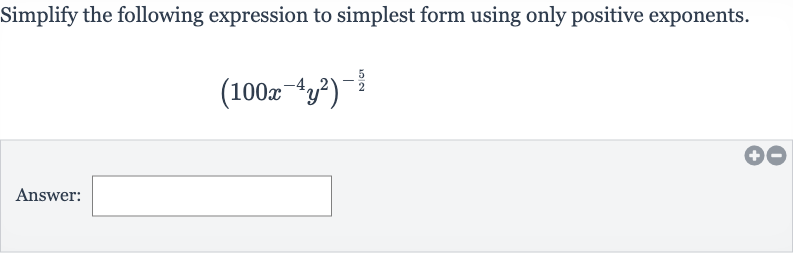
Q. Simplify the following expression to simplest form using only positive exponents.

\newline

\left(100 x^{-4} y^{2}\right)^{-\frac{5}{2}}

\newline

Answer:

Apply Negative Exponent Rule: Apply the negative exponent rule to the entire expression. $\newline$ The negative exponent rule states that $a^{-n} = \frac{1}{a^n}$ . We will apply this rule to the entire expression $(100x^{-4}y^{2})^{-\frac{5}{2}}$ .
Rewrite with Positive Exponents: Rewrite the expression with positive exponents by taking the reciprocal. $\newline$ $(100x^{-4}y^{2})^{-(5)/(2)}$ becomes $1/((100x^{-4}y^{2})^{5/2})$ .
Apply Power to Each Factor: Apply the power to each factor inside the parentheses. $\newline$ When raising a product to a power, we raise each factor to that power. So, we have: $\newline$ $\frac{1}{(100^{\frac{5}{2}})(x^{-4 \cdot \frac{5}{2}})(y^{2 \cdot \frac{5}{2}})}$ .
Simplify Each Factor: Simplify each factor separately. $\newline$ $100^{\frac{5}{2}}$ is the square root of $100$ raised to the $5^{\text{th}}$ power, which is $10^5$ . $\newline$ $x^{(-4 \cdot \frac{5}{2})}$ simplifies to $x^{-10}$ . $\newline$ $y^{(2 \cdot \frac{5}{2})}$ simplifies to $y^5$ . $\newline$ So, we have $\frac{1}{(10^5)(x^{-10})(y^5)}$ .
Rewrite $x^{-10}$ : Rewrite $x^{-10}$ with a positive exponent. $\newline$ $x^{-10}$ can be rewritten as $\frac{1}{x^{10}}$ using the negative exponent rule. $\newline$ So, we have $\frac{1}{(10^5)(\frac{1}{x^{10}})(y^5)}$ .
Combine Denominator Terms: Combine the terms in the denominator. $\newline$ When we multiply fractions, we multiply the numerators and the denominators separately. So, we have: $\newline$ $\frac{1}{\left(\frac{10^5}{x^{10}y^5}\right)}$ .
Simplify Expression: Simplify the expression. $\newline$ Since we have a fraction within a fraction, we can simplify by multiplying by the reciprocal of the denominator: $\newline$ $\frac{1}{\left(\frac{10^5}{x^{10}y^5}\right)} = \frac{x^{10}}{10^5 \cdot y^5}$ .