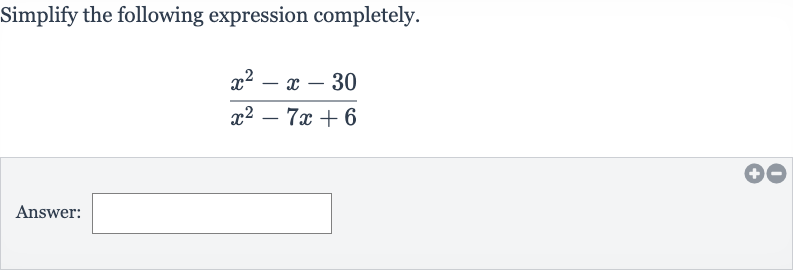
Simplify the following expression completely. (x^(2)-x-30)/(x^(2)-7x+6) Answer:

Question

Simplify the following expression completely.  (x^(2)-x-30)/(x^(2)-7x+6) Answer:

Bytelearn · Accepted Answer

Factor Numerator: First, we need to factor both the numerator and the denominator of the expression. Let's start with the numerator: x^2 - x - 30. We are looking for two numbers that multiply to -30 and add up to -1 (the coefficient of x). The numbers -6 and 5 satisfy these conditions because -6 * 5 = -30 and -6 + 5 = -1. So we can factor the numerator as (x - 6)(x + 5).Factor Denominator: Now, let's factor the denominator: x^2 - 7x + 6. We are looking for two numbers that multiply to 6 and add up to -7. The numbers -6 and -1 satisfy these conditions because -6 * -1 = 6 and -6 - 1 = -7. So we can factor the denominator as (x - 6)(x - 1).Cancel Common Factor: With both the numerator and denominator factored, the expression is now: ((x - 6)(x + 5)) / ((x - 6)(x - 1)). We can see that (x - 6) is a common factor in both the numerator and the denominator.Simplify Expression: We can cancel out the common factor (x - 6) from both the numerator and the denominator. This leaves us with (x + 5) / (x - 1).Final Simplified Form: The expression (x + 5) / (x - 1) is already in its simplest form. There are no common factors left to cancel, and both the numerator and the denominator are in their simplest form.

Simplify the following expression completely. $\newline$ $\frac{x^{2}-x-30}{x^{2}-7 x+6}$ $\newline$ Answer:

Full solution

More problems from Write variable expressions for arithmetic sequences

Related topics

Simplify the following expression completely.\newlinex2−x−30x2−7x+6 \frac{x^{2}-x-30}{x^{2}-7 x+6} x2−7x+6x2−x−30​\newlineAnswer:

Simplify the following expression completely. $\newline$ $\frac{x^{2}-x-30}{x^{2}-7 x+6}$ $\newline$ Answer: