AI tutor
Full solution
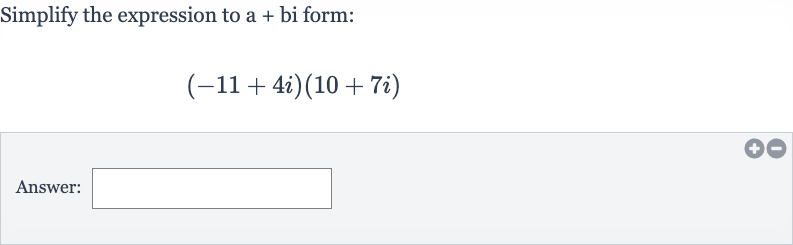
Q. Simplify the expression to a + bi form:Answer:
- Multiply Real Parts: We need to multiply two complex numbers and using the distributive property (also known as the FOIL method for binomials).
- Multiply Real and Imaginary: First, we multiply the real parts: .
- Combine Real and Imaginary: Next, we multiply the real part of the first complex number by the imaginary part of the second: .
- Final Expression: Then, we multiply the imaginary part of the first complex number by the real part of the second: .
- Final Expression: Then, we multiply the imaginary part of the first complex number by the real part of the second: .Finally, we multiply the imaginary parts: . Since , this becomes .
- Final Expression: Then, we multiply the imaginary part of the first complex number by the real part of the second: .Finally, we multiply the imaginary parts: . Since , this becomes .Now, we combine all the parts: real with real and imaginary with imaginary.Real: .Imaginary: .
- Final Expression: Then, we multiply the imaginary part of the first complex number by the real part of the second: .Finally, we multiply the imaginary parts: . Since , this becomes .Now, we combine all the parts: real with real and imaginary with imaginary.Real: .Imaginary: .The expression in form is: .
More problems from Write a quadratic function from its x-intercepts and another point
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help