AI tutor
Full solution
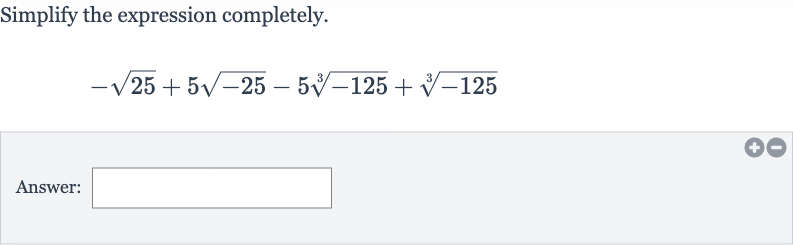
Q. Simplify the expression completely.Answer:
- Simplify : First, let's simplify each term in the expression separately. simplifies to because the square root of is .
- Calculate : Next, involves the square root of a negative number, which is not a real number. It is an imaginary number. The square root of is , where is the imaginary unit. So, simplifies to .
- Evaluate : Then, simplifies to because the cube root of is . So, simplifies to .
- Find : Finally, simplifies to because the cube root of is .
- Combine simplified terms: Now, we combine all the simplified terms: .
- Combine real numbers: Combining the real numbers: .
- Finalize imaginary term: The imaginary term remains as is because there are no other imaginary terms to combine it with.
- Final simplified expression: The final simplified expression is .