AI tutor
Full solution
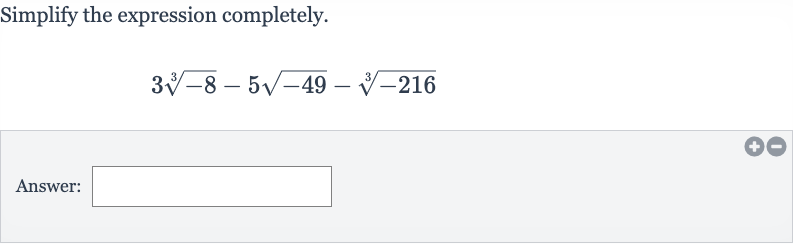
Q. Simplify the expression completely.Answer:
- Cube Root of : Simplify the cube root of . The cube root of is because . cube root of =
- Square Root of : Simplify the square root of \(-49").The square root of a negative number involves an imaginary unit i"), where \(i^\(2 = ").Square root of \(-49 = \sqrt{} \times \sqrt{} = i").\(5 \times \sqrt{} = \times i = i")
- Cube Root of : Simplify the cube root of . The cube root of is because . Cube root of =
- Combine Results: Combine the results from steps , , and .The expression now becomes .Combine the real numbers: The imaginary part remains unchanged: The simplified expression is .