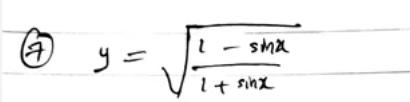
simplify and find the differentiation. (7) y=sqrt((1-sin x)/(1+sin x))

Question

simplify and find the differentiation. (7)  y=sqrt((1-sin x)/(1+sin x))

Bytelearn · Accepted Answer

Rewrite Function: Rewrite the function in a form that is easier to differentiate. We have y = sqrt((1-sin x)/(1+sin x)). To differentiate this, it's helpful to rewrite the square root as a power of 1/2. y = ((1-sin x)/(1+sin x))^(1/2)Apply Chain Rule: Apply the chain rule to differentiate the function. The chain rule states that the derivative of a composite function is the derivative of the outer function evaluated at the inner function times the derivative of the inner function. Let u = (1-sin x)/(1+sin x), then y = u^(1/2). We need to find dy/du and du/dx and then multiply them together to get dy/dx.Differentiate y with u: Differentiate y with respect to u. Using the power rule, the derivative of u^(1/2) with respect to u is (1/2)u^(-1/2). dy/du = (1/2)(1-sin x)/(1+sin x)^(-1/2)Differentiate u with x: Differentiate u with respect to x. u = (1-sin x)/(1+sin x) To differentiate this quotient, we use the quotient rule: (v' * u - u' * v) / v^2, where u = 1 - sin x and v = 1 + sin x. du/dx = (cos x * (1 + sin x) - (-cos x) * (1 - sin x)) / (1 + sin x)^2 Simplify the numerator: du/dx = (cos x + sin x * cos x + cos x - sin x * cos x) / (1 + sin x)^2 du/dx = (2 * cos x) / (1 + sin x)^2Multiply for dy/dx: Multiply dy/du by du/dx to get dy/dx. dy/dx = dy/du * du/dx dy/dx = ((1/2)(1-sin x)/(1+sin x)^(-1/2)) * ((2 * cos x) / (1 + sin x)^2) Simplify the expression: dy/dx = (cos x) / ((1+sin x)^(3/2))

Simplify and find the differentiation. $y=\sqrt{\frac{1-\sin x}{1+\sin x}}$

Full solution

More problems from Roots of rational numbers

Related topics

Simplify and find the differentiation. y=1−sin⁡x1+sin⁡x y=\sqrt{\frac{1-\sin x}{1+\sin x}} y=1+sinx1−sinx​​

Simplify and find the differentiation. $y=\sqrt{\frac{1-\sin x}{1+\sin x}}$