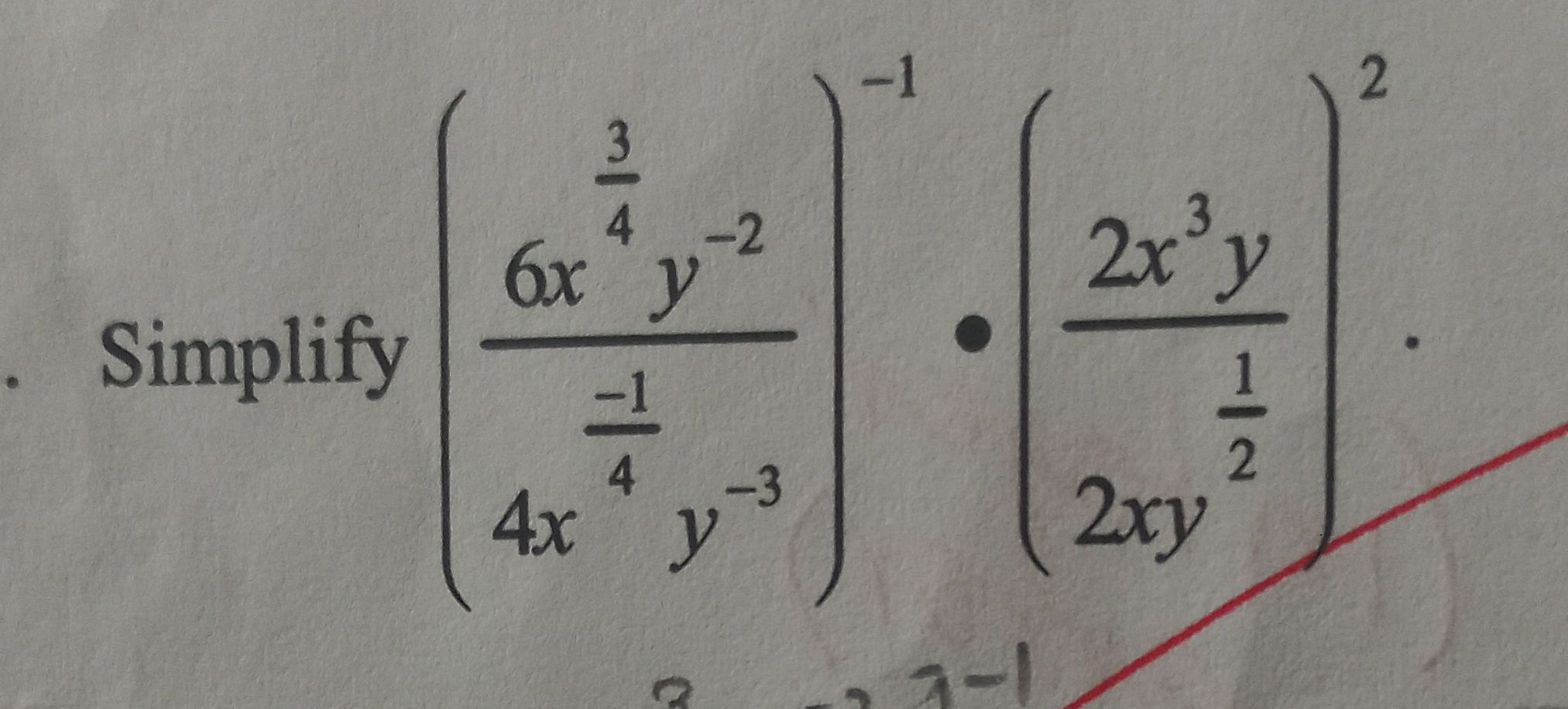Simplify ((6x^((3)/(4))y^(-2))/(4x^((-1)/(4))y^(-3)))^(-1)*((2x^(3)y)/(2xy^((1)/(2))))^(2)

Question

Simplify  ((6x^((3)/(4))y^(-2))/(4x^((-1)/(4))y^(-3)))^(-1)*((2x^(3)y)/(2xy^((1)/(2))))^(2)

Bytelearn · Accepted Answer

Rewrite Expression: Rewrite the expression to make it clearer. We have the expression: ((6x^(3/4)y^(-2))/(4x^(-1/4)y^(-3)))^(-1) * ((2x^3y)/(2xy^(1/2)))^2 First, we will simplify each part of the expression separately before combining them.Simplify First Part: Simplify the first part of the expression. We have: ((6x^(3/4)y^(-2))/(4x^(-1/4)y^(-3)))^(-1) To simplify, we will first deal with the exponents inside the parentheses before applying the outer exponent of -1.Combine Exponents: Combine the exponents of x and y inside the parentheses. We have: (6/4) * (x^(3/4) * x^(1/4)) * (y^(-2) * y^(3)) Simplify the coefficients: (6/4) = 3/2 Combine the exponents of x: x^(3/4 + 1/4) = x^(4/4) = x^1 = x Combine the exponents of y: y^(-2 + 3) = y^1 = y Now we have: (3/2) * x * yApply Outer Exponent: Apply the outer exponent of -1 to the simplified expression. We have: ((3/2) * x * y)^(-1) Taking the reciprocal of the expression: (2/3) * x^(-1) * y^(-1)Simplify Second Part: Simplify the second part of the expression. We have: ((2x^3y)/(2xy^(1/2)))^2 First, simplify the expression inside the parentheses. (2/2) * (x^3/x) * (y/y^(1/2)) The coefficients (2/2) cancel out to 1. Combine the exponents of x: x^(3-1) = x^2 Combine the exponents of y: y^(1-1/2) = y^(1/2) Now we have: (x^2 * y^(1/2))^2Apply Outer Exponent: Apply the outer exponent of 2 to the simplified expression. We have: (x^2 * y^(1/2))^2 Apply the exponent to both x and y: x^(2*2) * y^((1/2)*2) Simplify the exponents: x^4 * y^1 = x^4 * yCombine Simplified Expressions: Combine the simplified expressions from Step 4 and Step 6. We have: (2/3) * x^(-1) * y^(-1) * x^4 * y Combine the exponents of x: x^(-1+4) = x^3 Combine the exponents of y: y^(-1+1) = y^0 = 1 (since any number to the power of 0 is 1) Now we have: (2/3) * x^3Write Final Expression: Write the final simplified expression. The final simplified expression is: (2/3) * x^3

Simplify $\left(\frac{6 x^{\frac{3}{4}} y^{-2}}{4 x^{\frac{-1}{4}} y^{-3}}\right)^{-1} \cdot\left(\frac{2 x^{3} y}{2 x y^{\frac{1}{2}}}\right)^{2}$

Full solution

More problems from Multiplication with rational exponents

Related topics

Simplify (6x34y−24x−14y−3)−1⋅(2x3y2xy12)2 \left(\frac{6 x^{\frac{3}{4}} y^{-2}}{4 x^{\frac{-1}{4}} y^{-3}}\right)^{-1} \cdot\left(\frac{2 x^{3} y}{2 x y^{\frac{1}{2}}}\right)^{2} (4x4−1​y−36x43​y−2​)−1⋅(2xy21​2x3y​)2

Simplify $\left(\frac{6 x^{\frac{3}{4}} y^{-2}}{4 x^{\frac{-1}{4}} y^{-3}}\right)^{-1} \cdot\left(\frac{2 x^{3} y}{2 x y^{\frac{1}{2}}}\right)^{2}$