Resourcesdown chevron
Testimonials
Plans
Sign in
Sign up
Resourcesright arrow
Testimonials
Plans
Bytelearn - cat image with glassesAI tutor

Welcome to Bytelearn!

Let’s check out your problem:

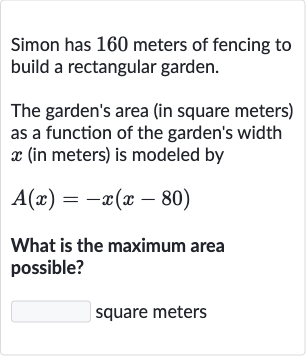
Simon has 160 meters of fencing to build a rectangular garden. The garden's area (in square meters) as a function of the garden's width x (in meters) is modeled by A(x)=-x(x-80) What is the maximum area possible? ◻ square meters

Simon has 160160 meters of fencing to build a rectangular garden. \newlineThe garden's area (in square meters) as a function of the garden's width xx (in meters) is modeled by\newlineA(x)=−x(x−80)A(x)=-x(x-80)\newlineWhat is the maximum area possible?\newline□\square square meters

View step-by-step helpView step-by-step help
Homeright chevron icon
Math Problemsright chevron icon
Algebra 2right chevron icon
Ratio and Quadratic equationright chevron icon

Full solution

Q. Simon has 160160 meters of fencing to build a rectangular garden. \newlineThe garden's area (in square meters) as a function of the garden's width xx (in meters) is modeled by\newlineA(x)=−x(x−80)A(x)=-x(x-80)\newlineWhat is the maximum area possible?\newline□\square square meters
  1. Perimeter Calculation: Simon has 160160 meters of fencing for a rectangular garden, which means the perimeter PP is 160160 meters. For a rectangle, P=2l+2wP = 2l + 2w, where ll is the length and ww is the width.
  2. Expressing Length in Terms of Width: Since the garden is rectangular, we can express the length in terms of the width as l=80−xl = 80 - x, because 2x+2(80−x)=1602x + 2(80 - x) = 160.
  3. Area Calculation: The area AA of the rectangle is given by A=l×wA = l \times w. Substituting l=80−xl = 80 - x, we get A(x)=x(80−x)A(x) = x(80 - x).
  4. Quadratic Function Analysis: The function A(x)=−x(x−80)A(x) = -x(x - 80) is a quadratic function that opens downwards (because of the negative sign), which means it has a maximum value at its vertex.
  5. Vertex Calculation: The vertex of a quadratic function in the form of f(x)=ax2+bx+cf(x) = ax^2 + bx + c is given by x=−b2ax = -\frac{b}{2a}. Here, a=−1a = -1 and b=80b = 80, so the x-coordinate of the vertex is x=−802∗(−1)=40x = -\frac{80}{2*(-1)} = 40.
  6. Maximum Area Calculation: Substitute x=40x = 40 into the area function to find the maximum area: A(40)=−40(40−80)=−40(−40)=1600A(40) = -40(40 - 80) = -40(-40) = 1600.

More problems from Ratio and Quadratic equation

Question
Solve by completing the square.\newlinem2−10m−29=0m^2 - 10m - 29 = 0\newlineWrite your answers as integers, proper or improper fractions in simplest form, or decimals rounded to the nearest hundredth.\newline`m` = ____ or `m` = _____
Get tutor helpright-arrow

Posted 8 months ago

Question
Find g(x) g(x) , where g(x) g(x) is the translation 5 5 units up of f(x)=x2 f(x)=x^2 .\newlineWrite your answer in the form a(x–h)2+k a(x–h)^2+k , where a a , h h , and k k are integers.\newlineg(x)= g(x)= ____
Get tutor helpright-arrow

Posted 7 months ago

Question
What is the range of this quadratic function?\newliney=x2−4x+4y = x^2 - 4x + 4\newlineChoices:\newline{y∣y≥2}\left\{y \mid y \geq 2\right\}\newline{y∣y≤0}\left\{y \mid y \leq 0\right\}\newline{y∣y≥0}\left\{y \mid y \geq 0\right\}\newlineall real numbers\text{all real numbers}
Get tutor helpright-arrow

Posted 5 months ago

Question
Write the equation of the parabola that passes through the points (1,0)(1,0), (2,0)(2,0), and (3,–16)(3,\text{–}16). Write your answer in the form y=a(x–p)(x–q)y = a(x – p)(x – q), where aa, pp, and qq are integers, decimals, or simplified fractions.\newline______
Get tutor helpright-arrow

Posted 5 months ago

Question
Complete the square. Fill in the number that makes the polynomial a perfect-square quadratic.\newlinef2+8f+_____f^2 + 8f + \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Solve for h h .\newlineh2+39h=0 h^2 + 39h = 0 \newline\newlineWrite each solution as an integer, proper fraction, or improper fraction in simplest form. If there are multiple solutions, separate them with commas.\newlineh= h = ____\newline
Get tutor helpright-arrow

Posted 5 months ago

Question
Write a quadratic function with zeros −9-9 and −7-7.\newlineWrite your answer using the variable xx and in standard form with a leading coefficient of 11.\newlinef(x)=_____f(x) = \_\_\_\_\_
Get tutor helpright-arrow

Posted 5 months ago

Question
Find the equation of the axis of symmetry for the parabola y=x2y = x^2. \newlineSimplify any numbers and write them as proper fractions, improper fractions, or integers.\newline‾\underline{\hspace{3cm}}
Get tutor helpright-arrow

Posted 4 months ago

Question
Find g(x)g(x), where g(x)g(x) is the translation 88 units up of f(x)=x2f(x) = x^2.\newlineWrite your answer in the form a(x–h)2+ka(x – h)^2 + k, where aa, hh, and kk are integers.\newlineg(x)=g(x) = ______\newline
Get tutor helpright-arrow

Posted 4 months ago

Question
Solve for xx.\newlinex2=1x^2 = 1\newline\newlineWrite your answer in simplified, rationalized form.\newlinex=x = ______ or x=x = ______\newline
Get tutor helpright-arrow

Posted 5 months ago

View all (23)

Related topics

Algebra - Order of OperationsAlgebra - Distributive Property`X` and `Y` AxesGeometry - Scalene TriangleCommon MultipleGeometry - Quadrant