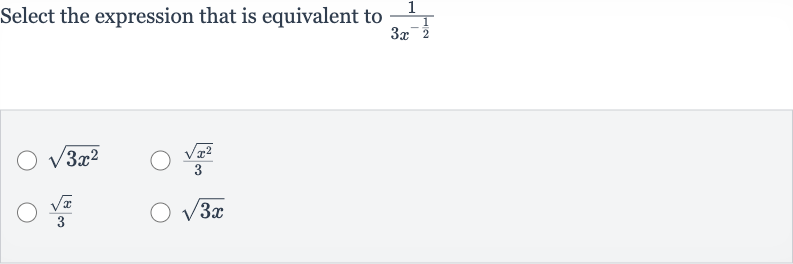Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

Select the expression that is equivalent to
(1)/(3x^(-(1)/(2)))

sqrt(3x^(2))

(sqrt(x^(2)))/(3)

(sqrtx)/(3)

sqrt(3x)

Select the expression that is equivalent to $\frac{1}{3 x^{-\frac{1}{2}}}$ $\newline$ $\sqrt{3 x^{2}}$ $\newline$ $\frac{\sqrt{x^{2}}}{3}$ $\newline$ $\frac{\sqrt{x}}{3}$ $\newline$ $\sqrt{3 x}$

View step-by-step help

View step-by-step help

Simplify radical expressions with root inside the root

Full solution

Q. Select the expression that is equivalent to

\frac{1}{3 x^{-\frac{1}{2}}}

\newline

\sqrt{3 x^{2}}

\newline

\frac{\sqrt{x^{2}}}{3}

\newline

\frac{\sqrt{x}}{3}

\newline

\sqrt{3 x}

Simplify Expression: First, let's simplify the given expression by removing the negative exponent. $\newline$ The negative exponent rule states that $a^{-n} = \frac{1}{a^n}$ . $\newline$ So, $\frac{1}{3x^{-\frac{1}{2}}}$ becomes $\frac{1}{3 \times \frac{1}{x^{\frac{1}{2}}}}$ .
Multiply Numerator and Denominator: Now, we simplify the expression by multiplying the numerator by the reciprocal of the denominator. $\newline$ This gives us $(1 \times x^{1/2})/3$ , which simplifies to $(x^{1/2})/3$ .
Convert to Square Root: The expression $(x^{\frac{1}{2}})/3$ is the same as $\frac{\sqrt{x}}{3}$ because $x^{\frac{1}{2}}$ is the square root of $x$ .
Compare with Options: Now, we compare the simplified expression $(\sqrt{x})/3$ with the given options to find the equivalent expression. $\newline$ The equivalent expression is $(\sqrt{x})/3$ .

More problems from Simplify radical expressions with root inside the root

Question

Find the real-number root.

\newline

\sqrt{16}

\newline

\newline

Write your answer in simplified form.

\newline

\_\_\_\_

Posted 3 months ago

Question

Find the real-number root.

\newline

\sqrt{\frac{9}{16}}

\newline

Simplify your answer and write it as a decimal or as a proper or improper fraction.

\newline

\_\_\_\_\_

Posted 3 months ago

Question

Find the real-number root using a calculator. Round your answer to the nearest thousandth.

\newline

\newline

Posted 3 months ago

Question

Multiply. Write your answer in simplest form.

\newline

\sqrt{273} \times \sqrt{42}

\newline

Posted 1 month ago

Question

\newline

\sqrt{\frac{63}{4}}

\newline

Posted 3 months ago

Question

Simplify. Rationalize the denominator.

\newline

\frac{-4}{9 - \sqrt{2}}

\newline

Posted 3 months ago

Question

z

\newline

16 = \sqrt{z}

\newline

z =

Posted 3 months ago

Question

Simplify the radical expression.

\sqrt{14x^{14}}

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

Posted 3 months ago

Question

Simplify the radical expression.

\newline

\sqrt{12x^{12}}

\newline

Write your answer in the form

A

\sqrt{B}

A\sqrt{B}

A

B

are constants or expressions in

x

. Use at most one radical in your answer, and at most one absolute value symbol in your expression for

A

\newline

\underline{\hspace{3cm}}

Posted 3 months ago

Question

What is the value of

\sin \left(\frac{5 \pi}{6}\right) ?

\newline

1

\newline

-\frac{\sqrt{3}}{2}

\newline

-\frac{\sqrt{2}}{2}

\newline

\frac{1}{2}

\newline

150

Posted 4 months ago