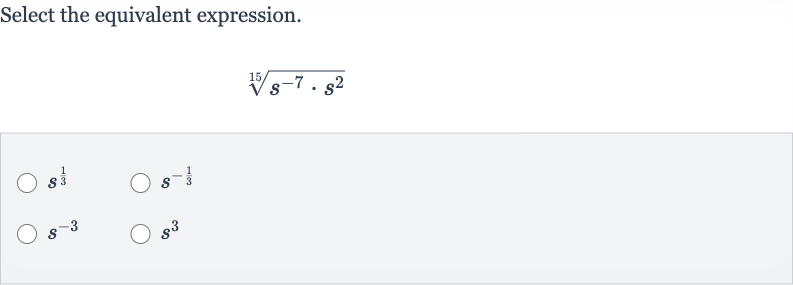AI tutor
Full solution
Q. Select the equivalent expression.
- Simplify Exponent Expression: Simplify the expression inside the root.We have the expression . To simplify the expression inside the root, we use the property of exponents that states when multiplying like bases, we add the exponents.
- Apply Root: Apply the root to the simplified expression.Now we have . The root of can be written as , which simplifies to .
- Match to Options: Match the simplified expression to the given options.The expression matches one of the given options, which is .
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help