AI tutor
Full solution
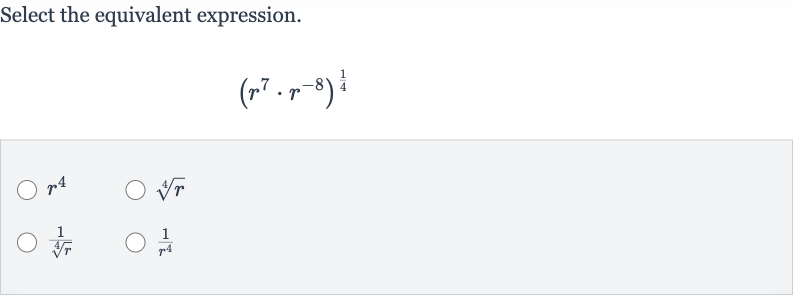
Q. Select the equivalent expression.
- Combine Exponents: Simplify the expression inside the parentheses by combining the exponents.When multiplying powers with the same base, you add the exponents.
- Apply Outer Exponent: Apply the outer exponent to the simplified base.Now we have . When raising a power to a power, you multiply the exponents.
- Rewrite Negative Exponent: Rewrite the negative exponent as a reciprocal.A negative exponent means that the base is on the wrong side of the fraction line, so we take the reciprocal and make the exponent positive.
- Recognize Fourth Root: Recognize that is the fourth root of . The expression can be rewritten using the radical notation for roots.
More problems from Multiplication with rational exponents
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help
QuestionGet tutor help