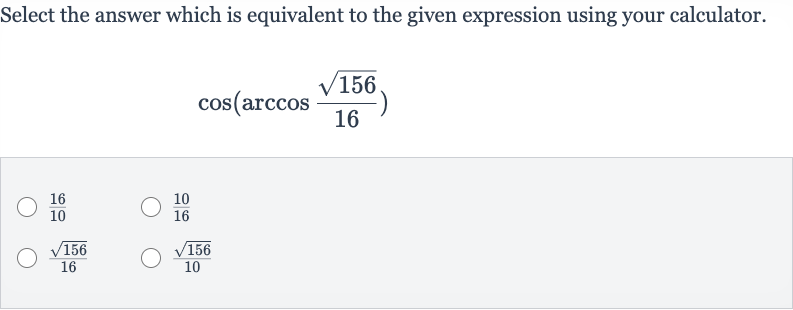Full solution
Q. Select the answer which is equivalent to the given expression using your calculator.
- Apply definition of arccosine: Apply the definition of the arccosine function.The arccos function is the inverse of the cosine function, which means that for any in the domain of arccos.
- Substitute given expression: Substitute the given expression into the identity.
- Check options for match: Check the given options to match the simplified expression.The simplified expression is , which is one of the given options.