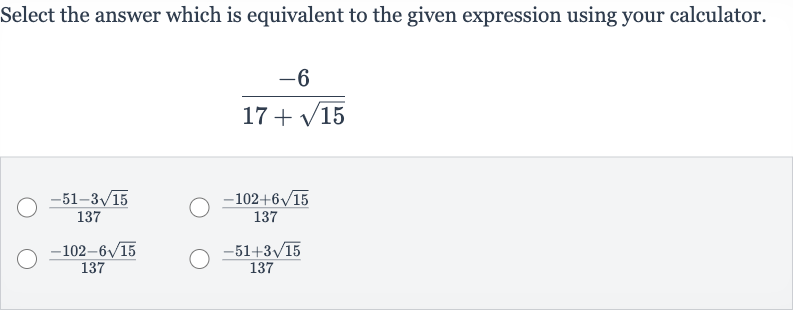Select the answer which is equivalent to the given expression using your calculator. (-6)/(17+sqrt15) (-51-3sqrt15)/(137) (-102+6sqrt15)/(137) (-102-6sqrt15)/(137) (-51+3sqrt15)/(137)

Question

Select the answer which is equivalent to the given expression using your calculator.  (-6)/(17+sqrt15)  (-51-3sqrt15)/(137)  (-102+6sqrt15)/(137)  (-102-6sqrt15)/(137)  (-51+3sqrt15)/(137)

Bytelearn · Accepted Answer

Rationalize Denominator: To find the equivalent expression for the given problem, we need to rationalize the denominator of the fraction (-6)/(17+sqrt15). This involves multiplying the numerator and the denominator by the conjugate of the denominator, which is (17-sqrt15).Multiply by Conjugate: The conjugate of (17+sqrt15) is (17-sqrt15). We multiply the numerator and the denominator by this conjugate to rationalize the denominator. ((-6)/(17+sqrt15)) * ((17-sqrt15)/(17-sqrt15)) = (-6*(17-sqrt15))/((17+sqrt15)*(17-sqrt15))Perform Numerator Multiplication: Now we perform the multiplication in the numerator: -6 * 17 = -102 and -6 * (-sqrt15) = 6sqrt15. So the numerator becomes (-102 + 6sqrt15).Multiply Denominators: Next, we multiply the denominators using the difference of squares formula: (17+sqrt15)(17-sqrt15) = 17^2 - (sqrt15)^2 = 289 - 15 = 274.Divide by 2: Now we have the fraction (-102 + 6sqrt15) / 274. To simplify this, we can divide both the numerator and the denominator by 2.Final Simplified Fraction: Dividing the numerator by 2 gives us (-102/2) + (6sqrt15/2) = -51 + 3sqrt15. Dividing the denominator by 2 gives us 274/2 = 137.The simplified fraction is now (-51 + 3sqrt15) / 137. This matches one of the answer choices provided.

Select the answer which is equivalent to the given expression using your calculator. $\newline$ $\frac{-6}{17+\sqrt{15}}$ $\newline$ $\frac{-51-3 \sqrt{15}}{137}$ $\newline$ $\frac{-102+6 \sqrt{15}}{137}$ $\newline$ $\frac{-102-6 \sqrt{15}}{137}$ $\newline$ $\frac{-51+3 \sqrt{15}}{137}$

Full solution

More problems from Sum of finite series starts from 1

Related topics