Resources

Resources

Bytelearn - cat image with glasses

AI tutor

Welcome to Bytelearn!

Let’s check out your problem:

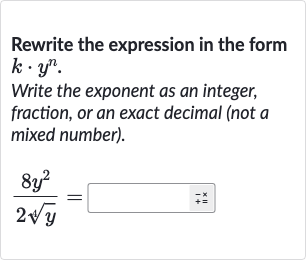
$Rewrite the expression in the form k*y^(n). Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). (8y^(2))/(2root(4)(y))=◻$

Rewrite the expression in the form $k \cdot y^{n}$ . $\newline$ Write the exponent as an integer, fraction, or an exact decimal (not a mixed number). $\newline$ $\frac{8 y^{2}}{2 \sqrt[4]{y}}=\square$

View step-by-step help

View step-by-step help

Evaluate variable expressions involving rational numbers

Full solution

Q. Rewrite the expression in the form

k \cdot y^{n}

.

\newline

Write the exponent as an integer, fraction, or an exact decimal (not a mixed number).

\newline

\frac{8 y^{2}}{2 \sqrt[4]{y}}=\square

Simplify Coefficients and Root: We are given the expression $(8y^{2})/(2\sqrt[4]{y})$ . The first step is to simplify the expression by dividing the coefficients and rewriting the root as an exponent. $\newline$ Divide $8$ by $2$ . $\newline$ $8 / 2 = 4$ $\newline$ Rewrite the fourth root of y as $y^{1/4}$ . $\newline$ Expression becomes: $4y^{2} \cdot y^{-1/4}$
Combine Like Terms: Now we need to combine the $y$ terms by adding their exponents, since they have the same base and are being multiplied. $\newline$ Use the rule: $y^{a} \cdot y^{b} = y^{a+b}$ $\newline$ Add the exponents $2$ and $-\frac{1}{4}$ . $\newline$ $2 + (-\frac{1}{4}) = 2 - \frac{1}{4} = \frac{8}{4} - \frac{1}{4} = \frac{7}{4}$ $\newline$ Expression becomes: $4y^{\frac{7}{4}}$

More problems from Evaluate variable expressions involving rational numbers

Question

Evaluate the expression for

j = 7

\newline

2j =

Posted 9 months ago

Question

Evaluate the expression for

y = 4

z = 5

\newline

yz =

Posted 8 months ago

Question

Evaluate the expression for

t = 0

\newline

|-4 - t| =

Posted 9 months ago

Question

8

pictures. Belle drew

p

fewer pictures than Dale. Choose the expression that shows how many pictures Belle drew.

\newline

\newline

8

\newline

p - 8

\newline

8 + p

\newline

8 - p

Posted 9 months ago

Question

Write an expression for the sequence of operations described below. subtract

5

6

w

to the result Do not simplify any part of the expression. ______

Posted 9 months ago

Question

Write an expression for the operation described below.

\newline

4

a

\newline

Posted 9 months ago

Question

12

cookies. Alice baked

n

fewer cookies than John.

\newline

Choose the expression that shows how many cookies Alice baked.

\newline

\newline

12

\newline

n - 12

\newline

12 + n

\newline

12 - n

Posted 10 months ago

Question

15

books. Lucas read

b

fewer books than Emma. Choose the expression that shows how many books Lucas read.

\newline

\newline

15

\newline

b - 15

\newline

15 + b

\newline

15 - b

Posted 10 months ago

Question

10

miles. Sarah ran

m

fewer miles than Max. Choose the expression that shows how many miles Sarah ran.

\newline

\newline

(A)10

\newline

(B)m - 10

\newline

(C)10 + m

\newline

(D)10 - m

Posted 10 months ago

Question

20

poems. Fiona wrote

p

fewer poems than Carlos. Choose the expression that shows how many poems Fiona wrote.

\newline

\newline

20

\newline

p - 20

\newline

20 + p

\newline

20 - p

Posted 10 months ago

Related topics

Algebra - Order of Operations Algebra - Distributive Property `X` and `Y` Axes Geometry - Scalene Triangle Common Multiple Geometry - Quadrant