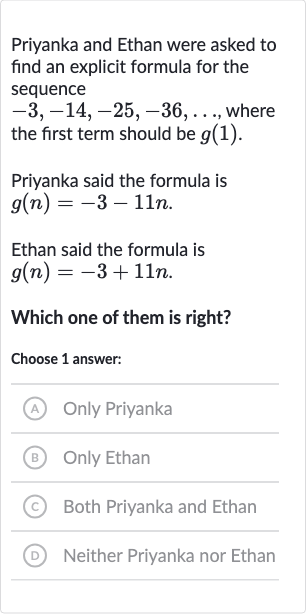
Priyanka and Ethan were asked to find an explicit formula for the sequence $-3,-14,-25,-36, \ldots$ , where the first term should be $g(1)$ . $\newline$ Priyanka said the formula is $g(n)=-3-11 n$ . $\newline$ Ethan said the formula is $g(n)=-3+11 n$ . $\newline$ Which one of them is right? $\newline$ Choose $1$ answer: $\newline$ (A) Only Priyanka $\newline$ (B) Only Ethan $\newline$ (C) Both Priyanka and Ethan $\newline$ (D) Neither Priyanka nor Ethan

Full solution

Q. Priyanka and Ethan were asked to find an explicit formula for the sequence

-3,-14,-25,-36, \ldots

, where the first term should be

g(1)

\newline

Priyanka said the formula is

g(n)=-3-11 n

\newline

Ethan said the formula is

g(n)=-3+11 n

\newline

Which one of them is right?

\newline

Choose

1

answer:

\newline

(A) Only Priyanka

\newline

(B) Only Ethan

\newline

\newline

(D) Neither Priyanka nor Ethan

Analyze Sequence Pattern: To determine the correct formula, we need to analyze the pattern of the sequence. We will look at the difference between consecutive terms to find the common difference.
Calculate Common Difference: The difference between the first and second term is $-14 - (-3) = -11$ . The difference between the second and third term is $-25 - (-14) = -11$ . The difference between the third and fourth term is $-36 - (-25) = -11$ . This shows that the sequence is arithmetic with a common difference of $-11$ .
Write Explicit Formula: Now we will use the common difference to write the explicit formula. The $n$ th term of an arithmetic sequence can be found using the formula $g(n) = a + (n - 1)d$ , where $a$ is the first term and $d$ is the common difference.
Substitute Values: Substituting the values we have, $a = -3$ (the first term) and $d = -11$ (the common difference), into the formula, we get $g(n) = -3 + (n - 1)(-11)$ .
Simplify Formula: Simplifying the formula, we get $g(n) = -3 - 11(n - 1)$ . Expanding the formula, we get $g(n) = -3 - 11n + 11$ .
Combine Like Terms: Further simplifying, we combine like terms to get $g(n) = -3 - 11n + 11 = -11n + 8$ .